THERMODYNAMICS
It is the branch of chemistry which deals with the energy changes taking place during physical and chemical changes.
SYSTEM
A system is that part of universe which is under investigation .
SURROUNDINGS
The part of the universe other than the system is known as surroundings.
Thus, Universe = System + Surroundings
In simple case surroundings implies air or water both.
TYPES OF SYSTEM
- Open system - A system which can exchange matter as well as energy with surroundings.
- Closed system – A system which can exchange energy and not matter with surroundings.
- Isolated system - A system which can exchange neither matter nor energy with surroundings.
- Homogeneous system – A system consisting of one phase only e.g. pure solid, a liquid or a mixture of gases.
- Heterogeneous system – It may consists of two or more phases e.g. a solid in contact with liquid etc.
STATE OF SYSTEM
The condition of existence of a system when its macroscopic properties have definite values is known as state of system e.g. at 1 atm pressure H2O is (a) Solid below 0°C, (b) liquid between 0°C - 100°C and (c) gas above 100°C.
MACROSCOPIC PROPERTIES
The properties which arise out of collective behaviour of large number of chemical entities e.g. pressure, volume, temperature, composition, colour, refractive index etc.
EXTENSIVE PROPERTIES
The properties which depend upon the quantity of the substance or substances present in the system e.g. volume, enthalpy, free energy, entropy, heat capacity.
INTENSIVE PROPERTIES
The properties which are independent of the quantity of the substance present in the system e.g. density, molar volume, temperature, melting point, boiling point, freezing point etc.
Note – Any extensive property if expressed as per mole or per gm becomes intensive property e.g. mass and volume are extensive properties but density is an intensive property.
STATE VARIABLES AND STATE FUNCTIONS
These are the macroscopic properties of the system which change with the change in the state of system. They depend upon the initial and final state of system. They are temperature, pressure, volume, chemical composition, energy, entropy, free energy.
INTERNAL ENERGY, INHERENT ENERGY OR HIDDEN ENERGY (IE)
It is the sum of all types of energies (as given below) associated with a system or substance.
- Translational energy of the molecules, Et
- Rotational energy, Er
- Vibrational energy, Ev
- Electronic energy, Ee
- Nuclear energy, En
- Interaction energy of molecules, Ei
E = Et + Er + Ev + Ee + En + Ei
It is a state function, depends upon chemical nature of the substance, amount, temperature, pressure etc. Its absolute value cannot be determined but change during a chemical process can be determined.
Internal energy depends on temperature, pressure, volume and quantity of matter. In most stable form the internal energy of an element is zero.
INTERNAL ENERGY CHANGE ( E)
E)
It is the amount of heat evolved or absorbed when a chemical reaction is carried out at constant volume and constant temperature. It is measured in bomb calorimeter.
Where, C= Heat capacity of calorimeter
M = Molecular mass of the substance
W = Mass of the substance taken
MODES OF THE TRANSFER OF ENERGY
Exchange of energy between system and surroundings can take place by
(a) Heat (b) Work
(c) Electrical Energy (d) Radiations
EXPRESSION FOR PV WORK
The mathematical expression for pressure-volume work is 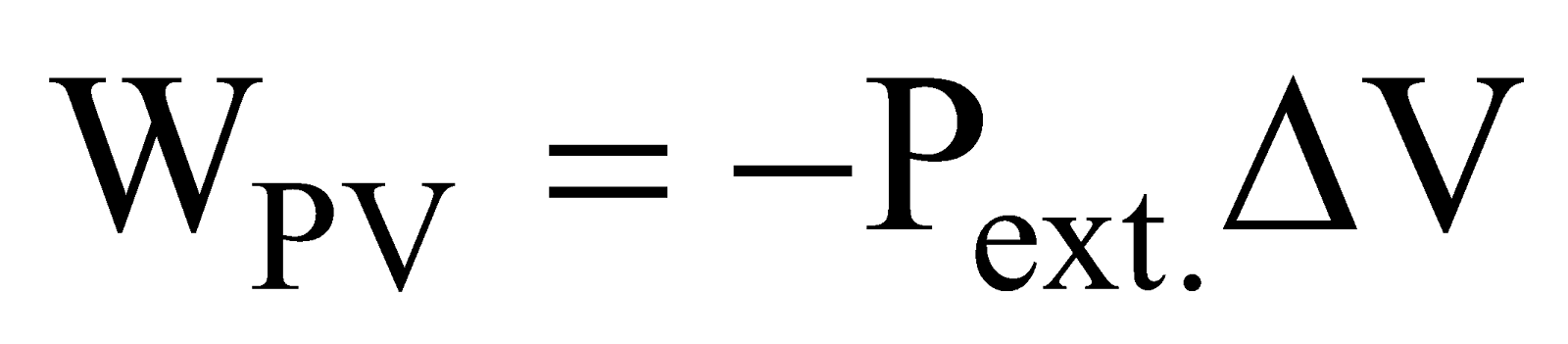
SIGN CONVENTIONS :
- Heat absorbed by the system is positive +ve
- Heat evolved by the system is negative –ve
- Work done on the system is positive +ve
- Work done by the system is negative –ve
PROCESSES AND THEIR TYPES
- Process - The operation by which a system changes from one state to another state is called process
- Reversible process - If the driving force is only infinitesimally greater than the opposing force and process can be reversed at any instant, it is known as reversible process.
- Irreversible process - It does not take place infinitesimally slow.
THERMODYNAMIC PROCESSES
- Isothermal process (
T = 0) : During each stage of process, the temperature remains constant. If the process is exothermic heat is given out to surroundings, If the process is endothermic heat is absorbed from surroundings.
- Adiabatic process (dq=0) : No heat leaves or enter the system, temperature of a system rises in exothermic process, temperature falls in endothermic process.
- Isobaric process
: Pressure remains constant, only volume changes.
- Isochoric process
: Volume remains constant but pressure changes.
- Cyclic process
: After undergoing a number of changes, the system returns to its original state. In cyclic process
THERMODYNAMIC EQUILIBRIUM
When there is no change in macroscopic properties with time. The types are :
- Thermal equilibrium - The temperature of the system remains constant throughout and no heat flows from one part to another part of system.
- Mechanical equilibrium - The pressure remains constant and no mechanical work is done by one part of system on another part.
- Chemical equilibrium - The composition of various phases remains the same.
FIRST LAW OF THERMODYNAMICS
- Law of equivalence of different forms of energies
- Energy can neither be created nor destroyed but can be transformed from one form to another.
- Total energy of universe is conserved (fixed, constant)
MATHEMATICAL EXPRESSION OF FIRST LAW
If q is the amount of heat absorbed by the system and w is the work done on the system, the change in internal energy,
If volume remains constant, no work is done then 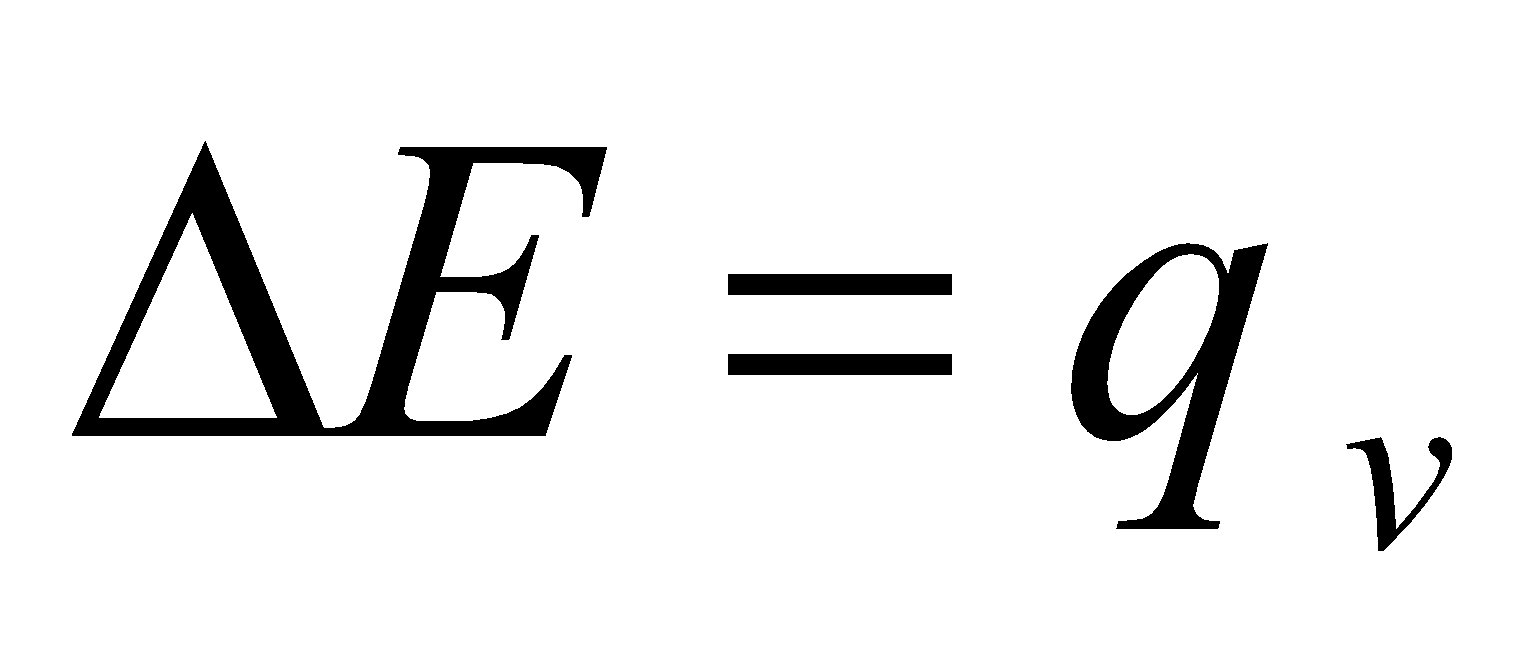
Note :- q and w are not state functions but the sum of the two (q + w) which represents is a state function
DISCUSSION OF EQUATION
(i) when 
If heat is absorbed by system, internal energy increases.
If heat is lost by system, internal energy decreases.
(ii) when 
If work is done on the system, internal energy increases.
If work is done by the system, internal energy decreases.
In adiabatic process work is done by the system at the expense of internal energy.
(iii) when 
Heat absorbed by the system is equal to work done by the system
If we write w = - q
If work is done on the system (when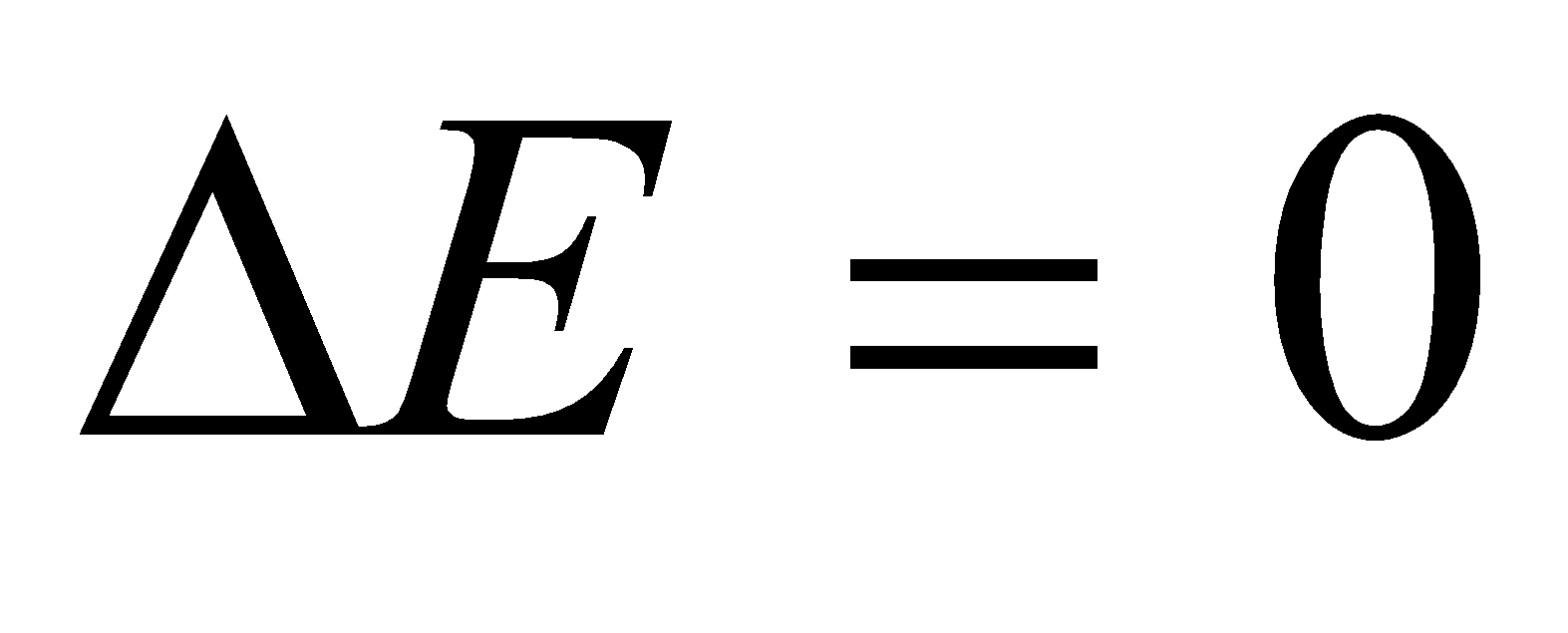 ) heat flows from system to surroundings.
) heat flows from system to surroundings.
(iv) Cyclic process, 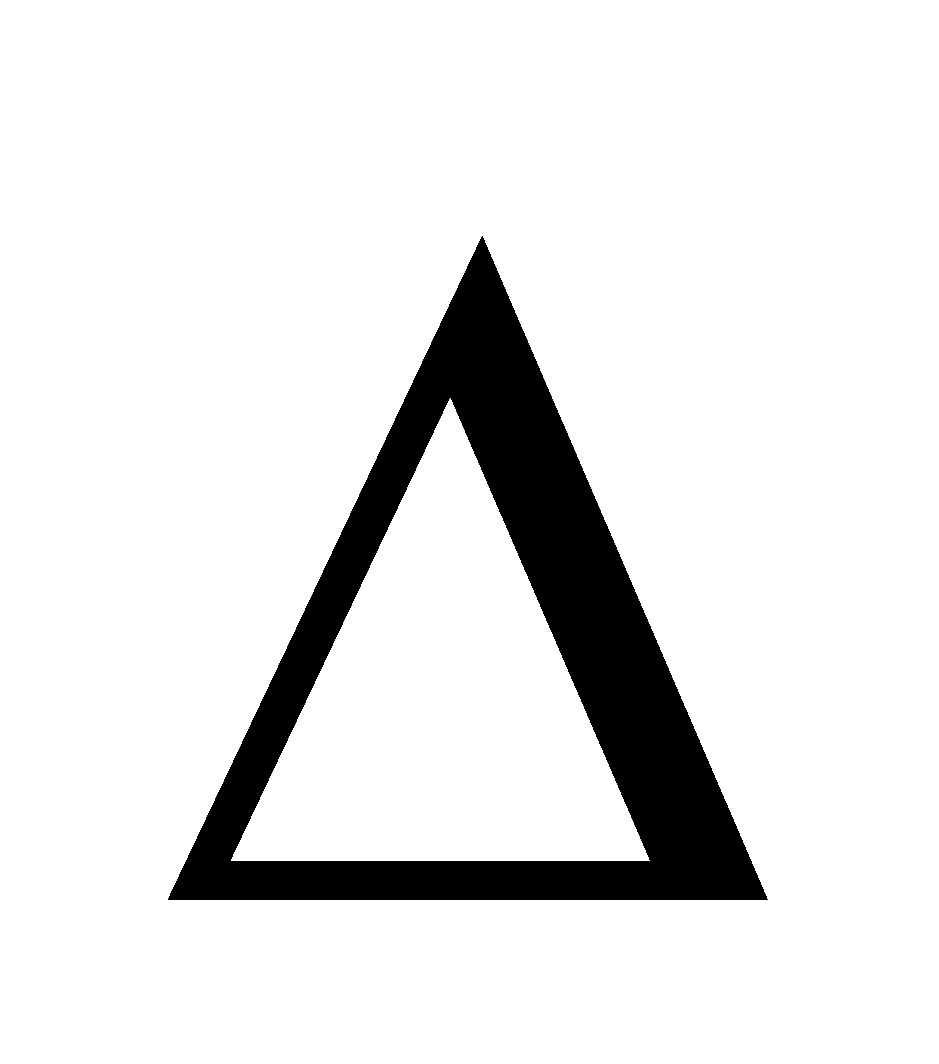 E = 0, q = –w
E = 0, q = –w
Work done by the system is equal to heat absorbed.
SIGNIFICANCE OF 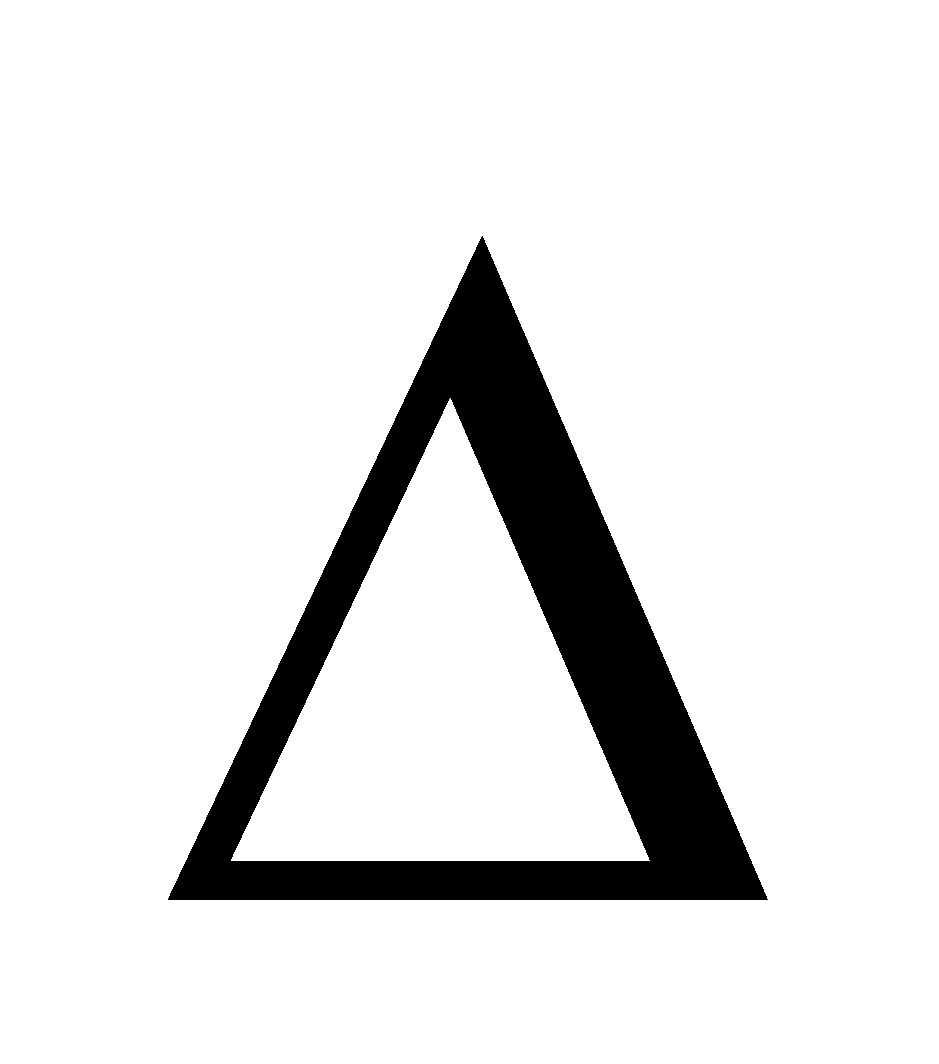 E
E
It represents the heat change taking place during the process occurring at constant volume and constant temperature.
qv = ΔE
ENTHALPY (H) AND ENTHALPY CHANGE ( H)
H)
Enthalpy is the heat content of the system and related to internal energy as follows.
H = E + PV
We can measure change in enthalpy and not absolute value of enthalpy. It is a state function.
Hproducts – H Reactants= HP – HR
If HP > HR, the change is endothermic. If HR > HP, the change is exothermic.
SIGNIFICANCE OF  H
H
The enthalpy H is given by
H = E + PV
the change in enthalpy is given by when pressure is kept constant
The enthalpy change represents the heat change taking place during the process occurring at constant pressure and constant temperature.
FACTORS AFFECTING THE  H
H
- Physical state of reactants and products
- Quantities of reactants and products
- Allotropic forms
- Temperature
- Conditions of constant P and Constant V at a particular temperature.
KIRCHOFF’S EQUATION
If reaction takes place at constant pressure, the variation of with temperature is given by this equation
If the reaction takes place at constant volume.
STANDARD ENTHALPY
The enthalpy change at the standard conditions i.e. at 1 atm pressure and 298K temperature is called standard enthalpy of the reaction and is denoted by 
RELATION BETWEEN  H AND
H AND  E:
E:
It is given by  , where
, where
 E AND
E AND  H FOR MONOATOMIC GAS :
H FOR MONOATOMIC GAS :
For such a gas
Internal Energy =  per mole and
per mole and
Enthalpy 
APPLICATIONS OF FIRST LAW OF THERMODYNAMICS
HESS’S LAW OF CONSTANT HEAT SUMMATION
Law is based upon the first law of thermodynamics and states that if a chemical change can be made to take place in two or more ways involving one or more steps, the net amount of heat change in the complete process is the same regardless of the method employed.
APPLICATIONS OF HESS’S LAW
- Calculation of heats of reactions
- Determination of heat changes of slow reactions
- Calculation of enthalpies of formation
- Calculation of bond energies
WORK DONE IN ISOTHERMAL REVERSIBLE EXPANSION (MAXIMUM WORK)
It is given by the expression
WORK DONE IN ADIABATIC REVERSIBLE EXPANSION
It is given by the expression
equal to and Cp – Cv = R
If we put the value of g we get the expression for workdone
Relation between temperature, volume and pressure in reversible adiabatic expansion
or T . P1–
. P1–  = const.
= const.
WORK DONE IN ADIABATIC IRREVERSIBLE EXPANSION
During expansion in vacuum
Pext = 0, work done = 0, also 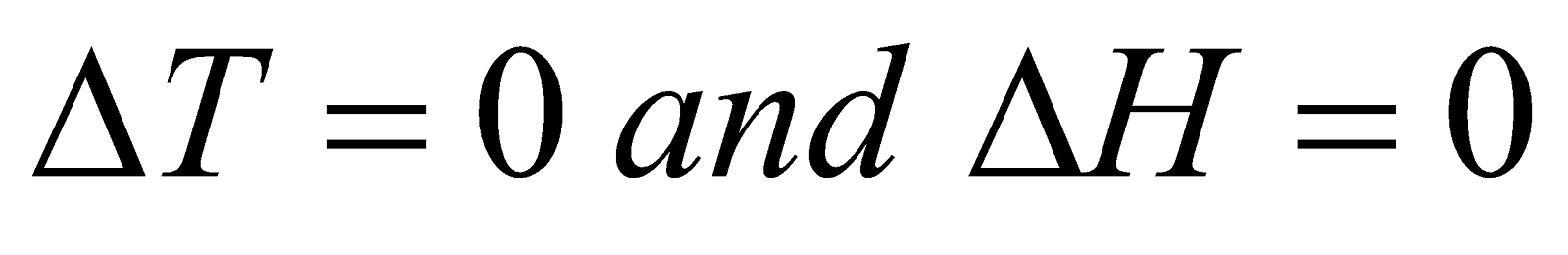
If  i.e. expansion work or work done by system.
i.e. expansion work or work done by system.
If 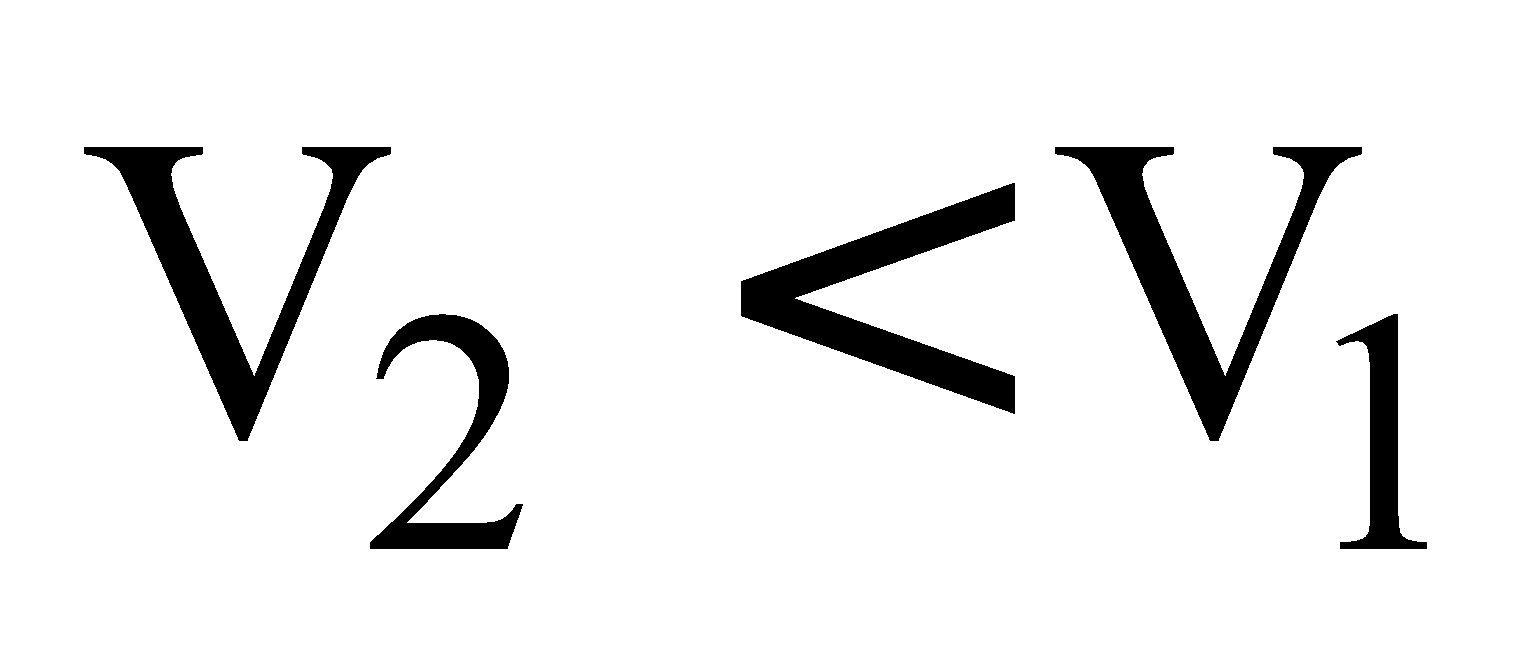 then Wirr is +ve i.e. compression work or work done on the system.
then Wirr is +ve i.e. compression work or work done on the system.
HEAT CAPACITY OF A SYSTEM
It is the amount of heat required to raise the temperature of the system by 1 degree (K or °C)
. 
In case of single substance, when m=1 g, C is the specific heat of system; when m = Mg or 1 mole, it is molar heat capacity. Since heat capacity varies with temperature its true value is given by
Heat capacity at constant volume 
Heat capacity at constant pressure 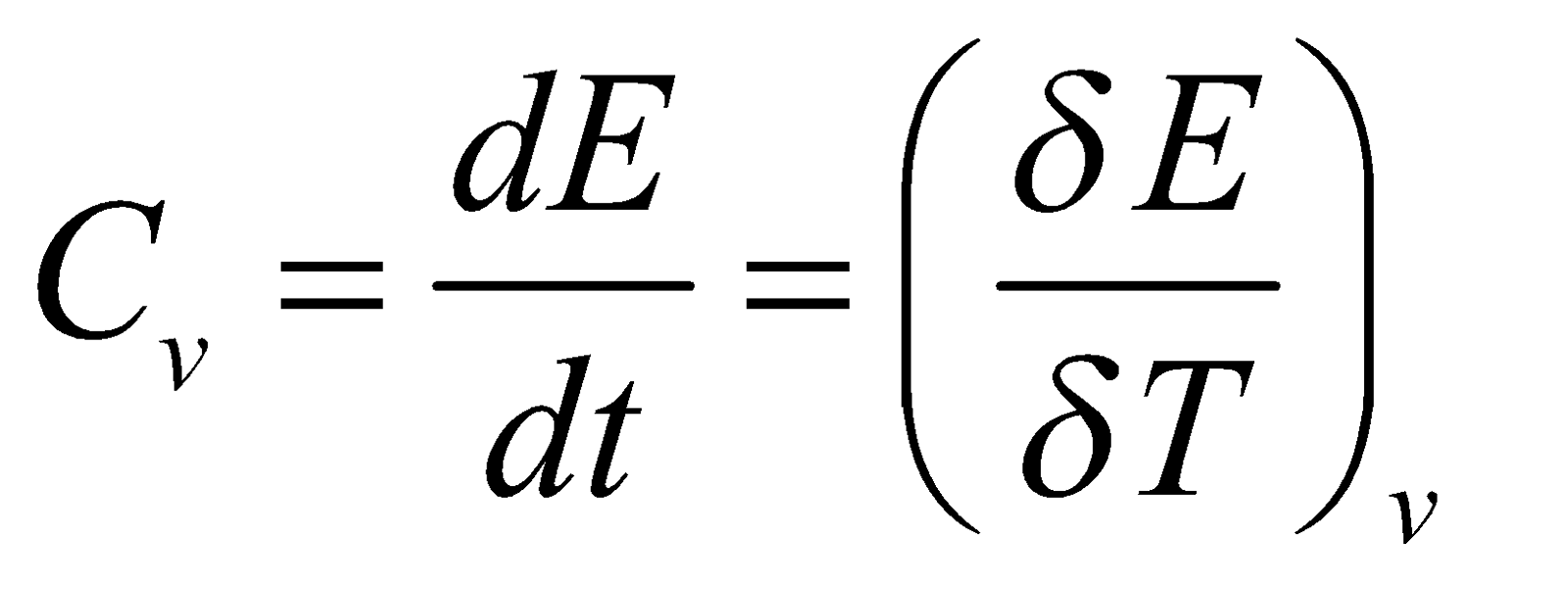
where  are specific heats at constant pressure and constant volume respectively
are specific heats at constant pressure and constant volume respectively
Cp and Cv are molar heat capacities at constant pressure and constant volume respectively
Dulong and Petit’s rule : Atomic mass × specific heat = 6.4
The rule is valid for solid elements except Be, B, Si and C.
Units : In SI units the specific heat or molar heat is expressed in terms of Joules ( 1 Cal = 4.184 J)
Molar heat capacity of a monoatomic gas at constant volume
Molar heat capacity of a monoatomic gas at constant pressure :
Molar heat capacity for polyatomic gas at constant volume :
Molar heat capacity for polyatomic gas at constant pressure :
For monoatomic gas X=0, for diatomic gas X=R and for triatomic gas 
LIMITATION OF FIRST LAW OF THERMODYNAMICS
It fails to predict the feasibility and the direction of the change.
SPONTANEOUS PROCESS
A process which has an urge or a natural tendency to occur either of its own or after proper initiation under a given set of conditions. It is irreversible process and may only be reversed by some external agents.
- Spontaneous processes that need no initiation
- Evaporation of water H2O (l) H2O (g)
- Dissolution of sugar
- Intermixing of gases
- Spontaneous processes that need initiation
- Electrolysis of water
It requires continuous supply of energy.
SECOND LAW OF THERMODYNAMICS
The entropy of the universe increases in every spontaneous (natural) change. The entropy of the universe is continuously increasing.
Other statements of second law of thermodynamics
- Clausius : Heat cannot itself pass from a colder body to a hotter body.
- Kelvin : It is impossible to get a continuous supply of work from a body or engine which can transfer heat from a single heat reservoir.
- Planck : It is impossible to construct a device which will work in a single complete cycle and convert heat into work without producing any change in the surroundings.
- All natural and spontaneous processes take place in one direction and thus are irreversible in nature.
- In a reversible process the entropy of the universe is constant. In an irreversible process the entropy of the universe increases.
Entropy (S)
It is a measure of degree of disorder or randomness in a system. More the disorder or randomness, the more is entropy.Heat added to a system increases randomness and hence the entropy. Heat added to a system at lower temperature causes greater randomness than the system at higher temperature. At higher temperature the system already has higher randomness. Thus entropy change is inversely proportional to temperature.
For a spontaneous process
Or  Suniverse >0
Suniverse >0
Units of entropy :
Jmol-1K-1; It is a state function. The change in entropy is represented by
qrev is the heat absorbed by the system in a reversible manner and isothermally.
ENTROPY CHANGE FOR AN IDEAL GAS UNDER DIFFERENT CONDITIONS: WITH CHANGE IN P, V AND T
For isothermal process 
For isobaric process 
For isochoric process 
GIBB’S FREE ENERGY (G)
It is the maximum amount of energy which can be converted into the useful work (other than PV work). It is related to enthalpy (H) and entropy (S) as follows.
HELMHOLTZ FREE ENERGY (WORK FUNCTION) (A)
The maximum work obtainable from a system is given by this energy.
A = E - TS ; E= Internal energy, S = Entropy, T= Temperature
CRITERIA FOR FEASIBILITY OR SPONTANEITY OF A PROCESS
Enthalpy or entropy alone cannot predict the spontaneity of a change. Gibb’s free energy is very useful factor for this
G = H – TS
Since, Heat lost by system = Heat gained by surroundings and vice versa.
T STotal = T
STotal = T Ssys –
Ssys –  Hsys
Hsys
= –( Hsys – T
Hsys – T Ssys)
Ssys)
= –( G)
G)
Hence,
- If
is negative, the process is spontaneous
- If
is zero, the process is in equilibrium
- If
is Positive the process does not occur
STANDARD FREE ENERGY CHANGE ( AND EQUILIBRIUM CONSTANT (K)
AND EQUILIBRIUM CONSTANT (K)
They are related as follows  2.303 RT log K
2.303 RT log K
FREE ENERGY CHANGE AND CELL POTENTIAL
VARIATION OF FREE ENERGY WITH TEMPERATURE AND PRESSURE IN A REVERSIBLE PROCESS
The equation is called the total differential equation.
(a) If temperature is kept constant dT=0 we have
(b) If pressure is kept constant dP = 0 we have
CHANGE IN FREE ENERGY FOR ISOTHERMAL PROCESS
CRITERIA OF FEASIBILITY OR SPONTANEITY IN TERMS OF :
- Entropy :
- Internal energy : When entropy and volume kept constant
If  , the process is irreversible and if
, the process is irreversible and if 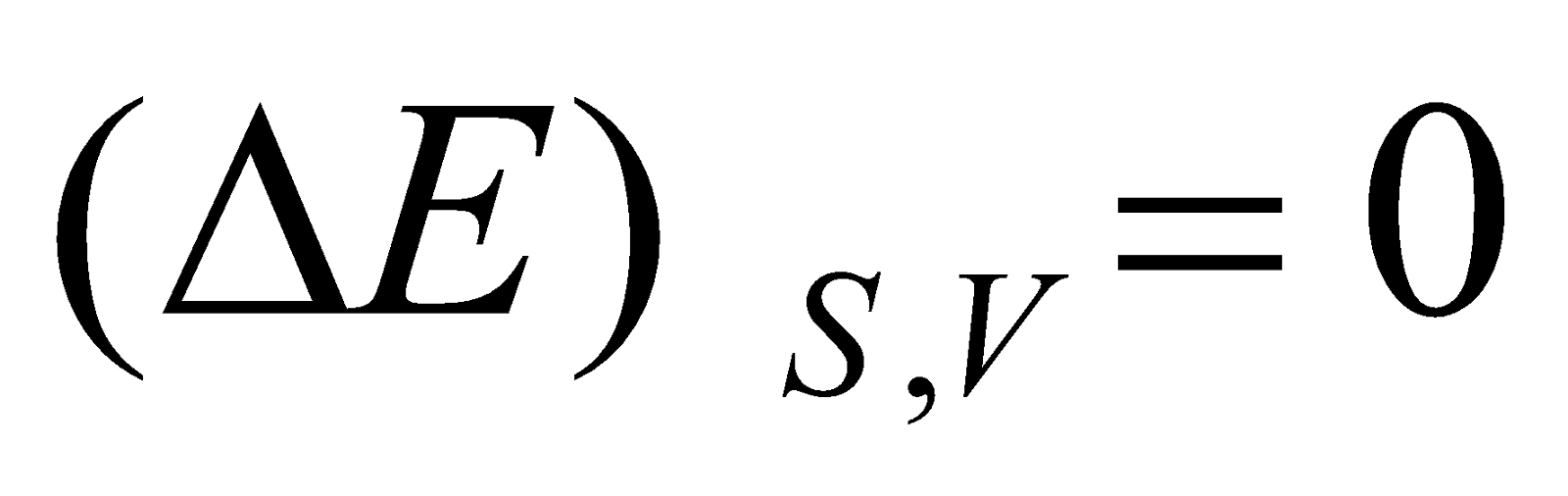 , the process is reversible.
, the process is reversible.
- Enthalpy (H) : When entropy and pressure are kept constant
, the process is irreversible and if
, the process is reversible.
- Work function (A) : When temperature and volume are kept constant
, the process is irreversible and if
, the process is reversible.
- Free energy (G) : When pressure and temperature are kept constant
, the process is irreversible and if
, the process is reversible.
Note : Criteria of feasibility or spontaneity in terms of free energy is most important since most of the processes are carried out at constant temperature and pressure.
ZEROTH LAW OF THERMODYNAMICS
If two bodies have separately equality of temperature with a third body, they also have equality of temperature with each other.
THIRD LAW OF THERMODYNAMICS
At absolute zero temperature, the entropy of a perfectly crystalline substance is taken as zero. This law was formulated by Nernst in 1906.
Calculation of absolute value of entropy:
Let S0 be the entropy of substance at 0 K and S be its entropy at T K.
Where Cp is the heat capacity of the substance at constant pressure. According to the third law
The value of the integral can be obtained from a plot of
CP / T Vs T. The area under the curve between 0 and T K gives the value of the integral and hence of S at temperature T.
CP / T Vs T. The area under the curve between 0 and T K gives the value of the integral and hence of S at temperature T.
CLAPEYRON - CLAUSIUS EQUATION
It gives the change in pressure dP accompanying change in temperature dT or vice versa for a system containing two phases of a pure substance in equilibrium, q is heat exchanged reversibly per mole of the substance during the phase transformation at temperature T.
- For water (liquid ) = water (vapour) we have
ΔHV = Molar heat of vaporisation
- For water (Solid, Ice) = water (liquid)
ΔHf = Molar heat of fusion of ice
- For solid = vapour equilibrium
ΔHs = Molar heat of sublimation
INTEGRATED FORM OF CLAPEYRON - CLAUSIUS EQUATION
For Liquid = gas system in Equilibrium
log 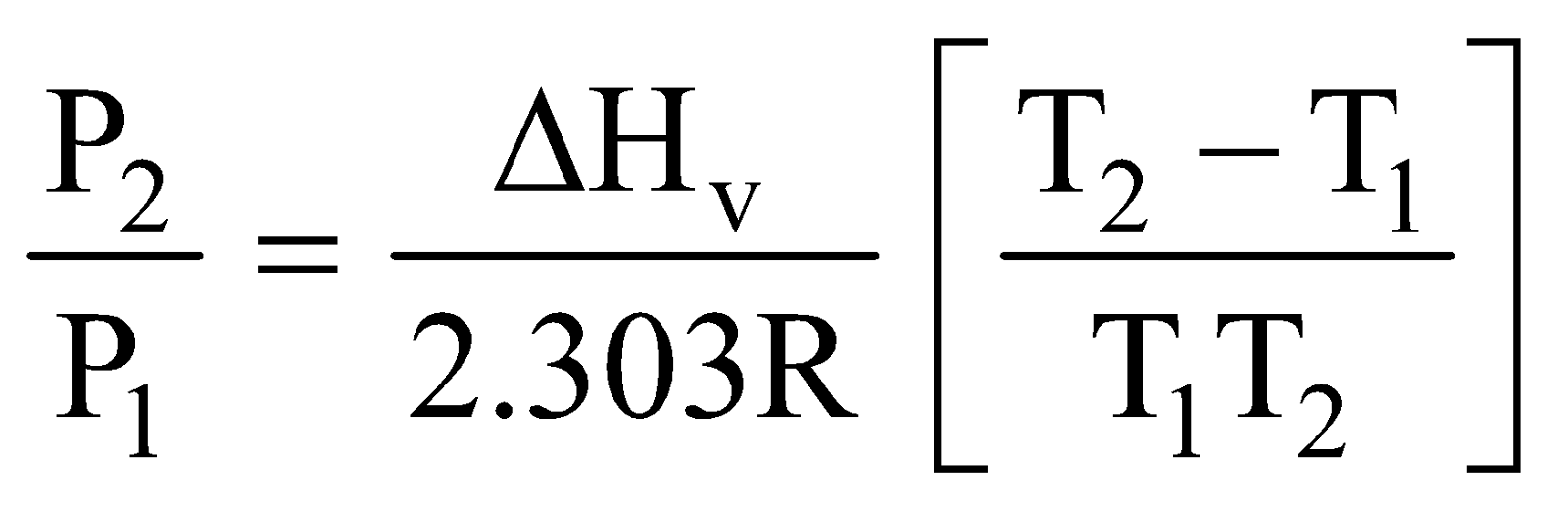
APPLICATIONS OF CLAPEYRON CLAUSIUS EQUATION
- Calculation of molar heat of vaporisation
- Effect of temperature on vapour pressure of a liquid
- Effect of pressure on boiling point
- Calculation of molal elevation constant (kb) and molal depression constant (kf) of a solvent.
lv= latent heat of vaporisation per gram of solvent
T= boiling point of pure solvent
T= freezing point of solvent
lf = latent heat of fusion per gram of solvent
HEAT ENGINE
Machine which converts heat into work is called heat engine.
EFFICIENCY OF HEAT ENGINE
The fraction of the heat absorbed, converted into work is called the efficiency of machine.
CARNOT CYCLE
It is a process where a system after undergoing a number of successive changes returns to its original state. It consists of four different operations.
- Isothermal expansion
- Adiabatic expansion
- Isothermal compression
- Adiabatic compression
EFFICIENCY OF CARNOT CYCLE OR ENGINE
Since  is always less than unity, hence efficiency is always less than unity
is always less than unity, hence efficiency is always less than unity
CARNOT THEOREM
Efficiency of reversible heat engine is independent of the nature of working substance and depends upon the temperature of source and sink.
or
All machines working reversibly between the same temperature of source and sink have same efficiency.
RESONANCE ENERGY
(Expected value of  ) – (Observed value of
) – (Observed value of  )
)
Example - Calculate the resonance energy of N2O from the following data
of N2O = 82 kJ mol–1, Bond energies of NN, N=N, O=O and N=O bands are 946, 418, 498 and 607 kJ mol–1 respectively
Sol. Calculated value of 
CALORIFIC VALUE
The heat liberated on burning unit mass of a fuel is called calorific value.
The more the calorific value the more the efficient is the fuel. Oxygen atoms present in a molecule reduce the calorific value.
The more the number of hydrogen atoms per carbon atom, the more is the calorific value.
TYPES OF REACTIONS AND CORRESPONDING ENTHALPY CHANGES
- Heat of reaction at constant volume and certain temperature
It is defined as the change in internal energy () of the system when requisite number of molecules of reactants react to form the products. (
) =
= heat of reaction at constant volume
- Heat of reaction at constant pressure and certain temperature
It is defined as the difference in enthalpies () of products and reactants
heat of reaction at constant pressure
- Relation between heat of reaction at constant volume (
) and at constant pressure (
)
- Sign of
and
: Negative sign of
or
shows that heat is evolved and reaction is exothermic while a positive sign of
and
shows that heat is absorbed and reaction is endothermic.
- Factors affecting the heat of reaction
- Physical state of the reactants and products
- Amount of the reactants
- Temperature
- Pressure
- Thermochemical equation
Stoichiometrically balanced, mentioning the physical state of the reactants and products and showing the values of and equation is called thermochemical equation
- Heat of formation (
) : It is defined as the enthalpy change
accompanying the formation of one mole of the compound from its elements.
- Standard heat of formation
: It is the enthalpy change accompanying the formation of one mole of a compound from its elements. All substances being in their standard states. The condition of standard state is 25ºC and 1 atm pressure.
- Heat of combustion : It is the change in enthalpy of the system, when 1 gram mole of the substance is completely oxidized
The combustion is always exothermic process.
- Application of heat of combustion
- Calorific value of fuel
- Calculation of heat of formation
- Constitution of organic compounds
- Heat of neutralization : It is defined as the enthalpy change (
) accompanying the neutralization of one gram equivalent of the acid by a base in dilute solution at that temperature.
Heat of neutralization of every strong acid by a strong base is identical. When acid or alkali is weak the heat of neutralization is different because the reaction involves the dissociation of the weak acid or the weak alkali.
Heat of neutralization of weak acids with NaOH at 25°C
- Heat of solution : The enthalpy change per mole of solute when it is dissolved in large excess of solvent (generally H2O) so that further dilution of the solution produces no heat exchange.
- Heat of dilution : The enthalpy change when a solution containing one mole of a solute is diluted from one concentration to another.
- Heat of hydration : The enthalpy change when one mole of a substance combines with the required number of moles of water to form a specified hydrate.
Heat of hydration = Heat of solution of the hydrated compound – Heat of solution of the unhydrated compound
- Heat of precipitation : The enthalpy change when one mole of a sparingly soluble substance precipitates on mixing dilute solutions of stable electrolytes is called heat of precipitation.
- Bond enthalpy : It is defined as the average amount of energy required to break all the bonds of a particular type in one mole of the substance. It is also known as bond energy and expressed in kJ mol–1.
In a diatomic molecule the bond energy and bond dissociation energy are the same.
In polyatomic molecules the bond dissociation energy is not the same for successive bonds though the bonds are of the same type.
- Use of bond enthalpy :
- Calculating standard enthalpy of reactions
= [ Total energy required for breaking the bonds] – [Energy given out in forming the bonds]
- Calculation of bond energy of some specific bond in the molecule
LAWS OF THERMOCHEMISTRY
- Lavosier and Laplace law : The heat evolved or absorbed in the course of a chemical reaction is equal to the heat absorbed or evolved when the reaction is reversed.
- Hess’s law of constant heat summation
TROUTON’S RULE
Fredrick Trouton (1884) found that for many liquids molar heat of vaporization in calories per mole divided by the normal boiling point in degree kelvin is a constant.
The quantity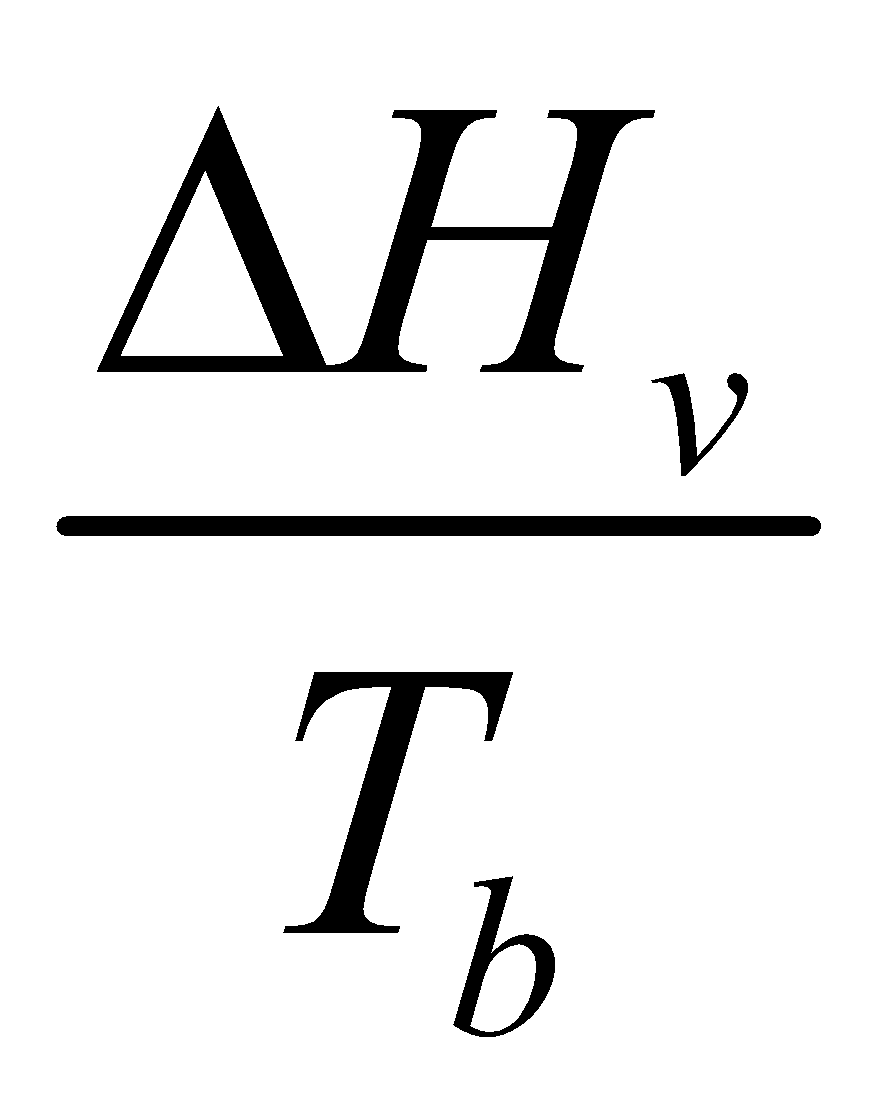 is also called entropy of vaporization
is also called entropy of vaporization  Sv.
Sv.
ENDOTHERMIC COMPOUNDS
The compounds having positive values of formation are called endothermic compounds. Ozone is an example.










