MAGNETISM AND MATTER
NATURAL MAGNET
A natural magnet is an ore of iron (Fe3O4) which
- attracts small pieces of iron, cobalt and nickel towards it.
- when suspended freely, comes to rest along north-south direction.
The magnets which are obtained artificially are called artificial magnets, e.g. a bar magnet, a magnetic needle, horse shoe magnet etc.
BAR MAGNET
A bar magnet consists of two equal and opposite magnetic poles separated by a small distance. Poles are not exactly at the ends. The shortest distance between two poles is called effective length (Le) and is less than its geometric length (Lg).
For bar magnet Le = 2l and Le =(5/6) Lg.
For semi-circular magnet Lg = πR and Le = 2R.
PROPERTIES OF MAGNETS
- Attractive property : A magnet attracts small pieces of iron, cobalt, nickel, etc. and other magnetic substances.
- Directive property : A freely suspended magnet aligns itself nearly in the geographical north-south direction.
- Law of magnetic poles : Like magnetic poles repel, and unlike magnetic poles attract each other.
According to Gauss’s theorem in magnetism, surface integral of magnetic field intensity over a surface (closed or open) is always zero i.e.

This theorem establishes that the poles always exist in equal and unlike pairs.
- Magnetic poles exist in pairs : Isolated magnetic poles do not exist. If we break a magnet into two pieces, we get two smaller dipole magnets.
- Repulsion is a sure test of magnetism.
MAGNETIC FIELD
The space around a magnet within which its influence can be experienced is called its magnetic field.
UNIFORM MAGNETIC FIELD
A uniform magnetic field is one where the strength of the magnetic field is the same at all points of the field. In a uniform field, all the magnetic lines of force are parallel to one another. But in non-uniform magnetic field the strength of magnetic field is not same at all points of the field and also the magnetic lines of force are not parallel.
ATOM AS A MAGNETIC DIPOLE
Every atom of a magnetic material behaves as a magnetic dipole, because electrons in the atom revolve around the nucleus. The magnetic moment M associated with an atomic dipole as
where n = 1, 2, 3 ..... denotes the no. of orbits and  . Least value of dipole moment of atom = 9.27 × 10–24 Am2.
. Least value of dipole moment of atom = 9.27 × 10–24 Am2.
Most of the magnetic moment is produced due to electron spin, the contribution of the orbital revolution is very small.
MAGNETIC LINES OF FORCE
Magnetic line of force is an imaginary curve the tangent to which at a point gives the direction of magnetic field at that point or the magnetic field line is the imaginary path along which an isolated north pole will tend to move if it is free to do so.
PROPERTIES OF MAGNETIC LINES OF FORCE
- Magnetic lines of force are hypothetical lines use to depict magnetic field in a region and understand certain phenomenon in magnetism.
- Tangent to field line at a point gives us the direction of magnetic field intensity
at that point. No two magnetic lines of force can intersect each other because magnetic field will have two directions at the point of intersection.
- Magnetic lines of force are continuous curve from north to south, outside the body of the magnet and from south to north inside the body of the magnet.
- The number of lines originating or terminating on a pole is proportional to its pole strength.
Magnetic flux = number of magnetic lines of force = µ0 × m
Where µ0 is number of lines associated with unit pole.
- The number of lines of force per unit area at a point gives magnitude of field at that point. The crowded lines show a strong field while distant lines represent a weak field.
- The magnetic lines of force have a tendency to contract longitudinally like a stretched elastic string producing attraction between opposite pole.
- The magnetic lines of force have a tendency to repel each other laterally resulting in repulsion between similar poles.
- The region of space with no magnetic field has no lines of force. At neutral point where resultant field is zero there cannot be any line of force.
SOME TERMS RELATED TO MAGNETISM
Magnetic poles : These are the regions of apparently concentrated magnetic strength where the magnetic attraction is maximum. It means that pole of a magnet is located not at a point but over a region. Magnetic poles exist in pairs. An isolated magnetic pole (north or south) does not exist. If a magnet is cut into two pieces, then instead of obtaining separate N-pole and S-pole, each of the two parts are found to behave as complete magnets.
Magnetic axis : The line passing through the poles of a magnet is called its magnetic axis.
Magnetic equator : The line passing through the centre of the magnet and at right angles of the magnetic axis is called the magnetic equator of the magnet.
Magnetic length : The shortest distance between the two poles of a magnet is called its magnetic length. It is less than the geometrical length of the magnet. This magnetic length is also called an effective length.
MAGNETIC MOMENT
The magnetic moment of a magnet in magnitude is equal to the product of pole strength with effective length (i.e. magnetic length). Its direction is along the axis of magnet from south pole to north pole.
If the same bar magnet is bent in a semicircle then
Net magnetic moment
Where m is pole strength, 2 is effective length and  is unit vector having a direction from S-pole to N-pole.
is unit vector having a direction from S-pole to N-pole.
The SI unit of  is A m2, which is equivalent to J/T.
is A m2, which is equivalent to J/T.
CIRCULAR CURRENT LOOP AS A MAGNET
A small plane loop of current behaves as a magnet with a definite dipole moment given by
where A is the area of the loop, I the current in the loop and is a unit vector perpendicular to the plane of the loop, and its direction is decided by the sense of flow of current I using the Fleming’s right hand rule.
RELATION BETWEEN MAGNETIC MOMENT AND ANGULAR MOMENTUM
Where q is the total charge on a body of mass m rotating about a fixed axis.
MAGNETIC MOMENT, POLE STRENGTH AND EFFECTIVE LENGTH WHEN A MAGNET IS CUT
COULOMB’S LAW OF MAGNETIC FORCE
It states that :
- The force of attraction or repulsion between two magnetic poles is directly proportional to the product of their pole strengths.
- The force of attraction or repulsion between two magnetic poles is inversely proportional to the square of the distance between them. This law is also known as inverse square law.
i.e., F ∝ m1m2 and F ∝ 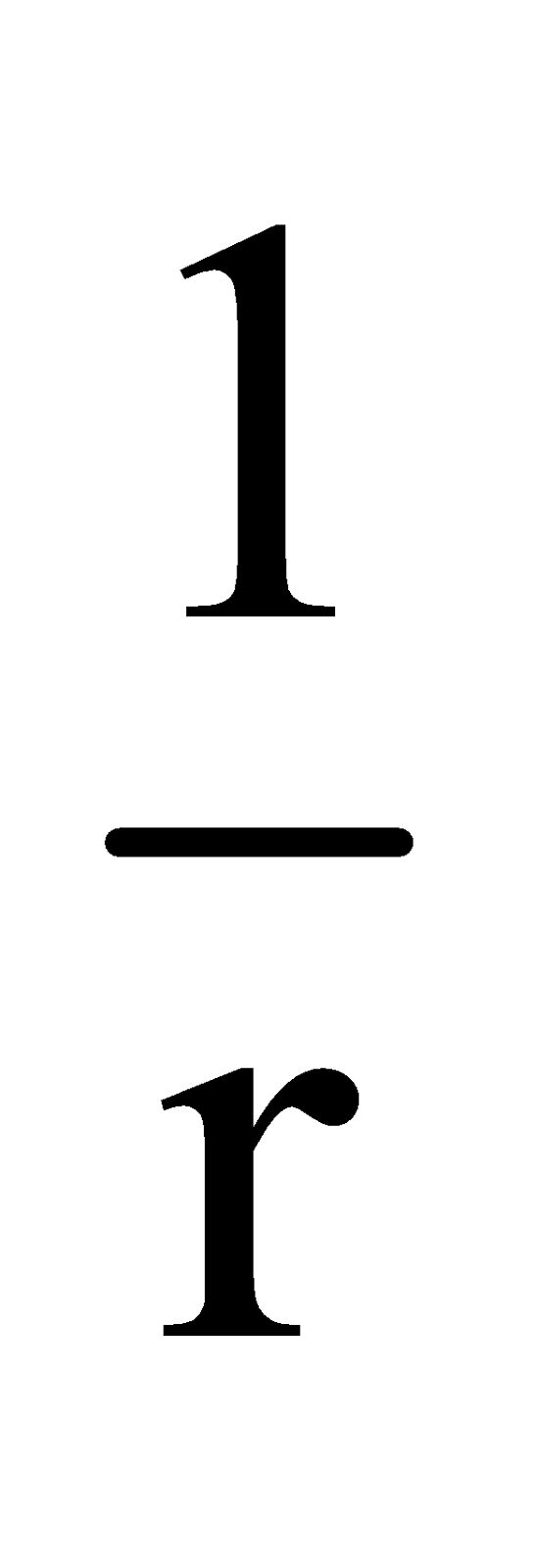
or, 
where m1 and m2 are the pole strengths of the two magnetic poles, r is the distance between them and μ0 is the permeability of free space.
Unit magnetic pole : A unit magnetic pole may be defined as the pole which when placed in vacuum at a distance of one metre from an identical pole, repels it with a force of 10–7 newton.
TORQUE ON A MAGNET IN A MAGNETIC FIELD
A magnet of dipole moment M suspended freely in a magnetic field B experiences a torque  given by
given by
where θ is the angle between  and
and 
It is clear from the expression that 
i.e., when dipole is perpendicular to field the torque is maximum and when they are parallel, the torque is minimum
(for θ = 0 or 180º →  ).
).
The net force acting on a bar magnet placed
- in a uniform magnetic field is zero
- in a non-uniform magnetic field is non-zero
Let the length of a bar magnet be 2l and pole strength be m, the magnetic field is B, and the angle between B and bar magnet is θ. Force on north pole is mB along the field and that on south pole is mB opposite to the field.
The torque of these two forces about O is
τ = 2mBlsinθ = MBsinθ
where M is the magnetic moment of the magnet. 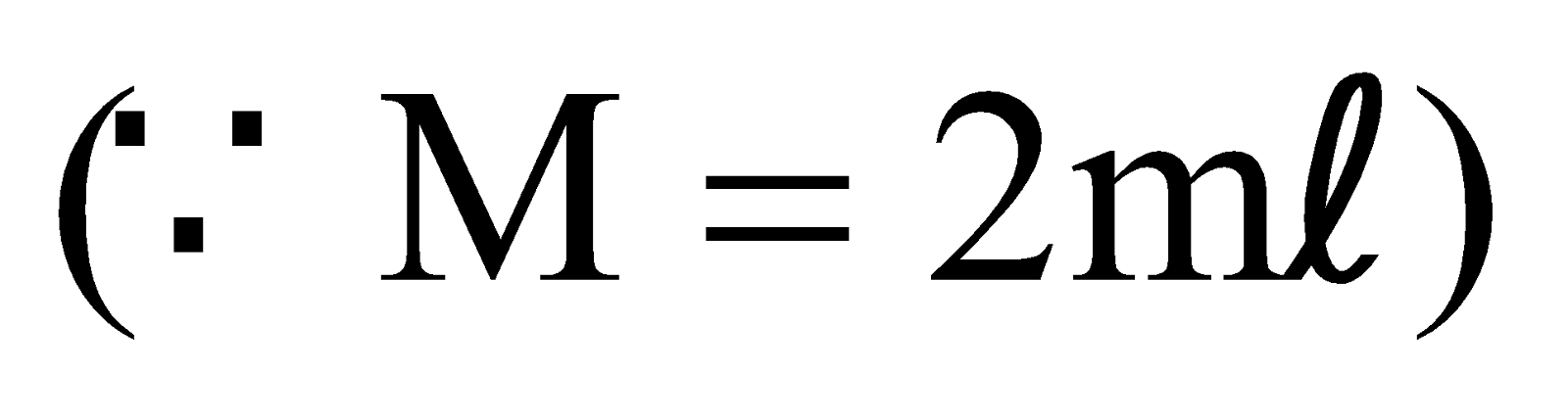
This torque tries to align the magnet with the field.
WORK DONE BY EXTERNAL AGENT IN ROTATING THE MAGNET
If an external agent rotates the magnet slowly, the agent has to exert a torque MBsinθ opposite to that exerted by the field.
Work done by the agent in changing the angle from θ to θ + dθ is dW = (MBsinθ) dθ
Wext is stored as potential energy of the field-magnet system. Thus
If we take 
POTENTIAL ENERGY
(i) When θ = 0, U = – MB (minimum PE)
(ii) When , U = 0
, U = 0
(iii) When , U = MB (maximum PE)
, U = MB (maximum PE)
WORK DONE IN ROTATING A UNIFORM MAGNETIC DIPOLE IN A MAGNETIC FIELD
Work done in deflecting the dipole through an angle θ is,
W = MB (1 – cos θ)
If θ = 0, cos θ = 1 then W = MB (1 – 1) = 0
If θ = 90°, cos θ = 0 then W = MB
If θ = 180°, cos θ = –1, then W = 2MB
GAUSS'S LAW IN MAGNETISM
The surface integral of magnetic field  over a closed surface S is always zero.
over a closed surface S is always zero.
Mathematically 
- Isolated magnetic poles do not exist is a direct consequence of gauss's law in magnetism.
- The total magnetic flux linked with a closed surface is always zero.
- If a number of magnetic field lines are leaving a closed surface, an equal number of field lines must also be entering the surface.
MAGNETIC FLUX
The magnetic flux through a given area may be defined as the total number of magnetic lines of force passing through this area. It is equal to the product of the normal components of the magnetic field B and the area over which it is uniform. In general,
Magnetic flux,  , where θ is angle between normal to the area dA with magnetic field B.
, where θ is angle between normal to the area dA with magnetic field B.
Magnetic flux linked with a closed surface is zero i.e., 
The S.Ι. Unit of magnetic flux is weber (Wb) : If a magnetic field of 1 tesla passes normally through a surface of area 1 square metre, then the magnetic flux linked with this surface is said to be 1 weber.
OSCILLATIONS OF A BAR MAGNET IN A MAGNETIC FIELD
A freely suspended magnet of magnetic moment M and of moment of inertia I oscillates simple harmonically in a magnetic field B with frequency
MAGNETIC FIELD DUE TO A BAR MAGNET
MAGNETIC FIELD INTENSITY B1 DUE TO A BAR MAGNET AT ANY POINT ON THE AXIAL LINE OF THE MAGNET IS
where d = distance of the point from the centre of the magnet.
The direction of B1 is along SN.
BN is magnetic field due to north pole, it is directed away from the magnet.
If d > > l, 
MAGNETIC FIELD INTENSITY (B2) DUE TO A BAR MAGNET AT ANY POINT ON THE EQUATORIAL LINE OF THE BAR MAGNET IS
The direction of B2 is along a line parallel to NS.
Now, 
Resultant field at P is, 
If d > > l, 
MAGNETIC FIELD AT ANY POINT HAVING POLAR COORDINATES (R, Θ) RELATIVE TO CENTRE OF MAGNET OR LOOP
MAGNETIC POTENTIAL
The magnetic potential at a point is defined as the work done in carrying a unit N-pole from infinity to that point against the field. It may also be defined as the quantity whose space rate of variation in any direction gives the intensity of the magnetic field.
i.e., 
MAGNETIC POTENTIAL DUE TO A POINT DIPOLE, AT A DISTANCE R FROM THE POLE OF STRENGTH M IS GIVEN BY
B due to a pole of Pole strength m at a distance r is given by 
Now V0 at A is the work done is bringing a unit pole from infinity to A.
MAGNETIC POTENTIAL DUE TO A MAGNETIC DIPOLE AT A POINT IN END-SIDE ON POSITION IS
 , where M = 2ml.
, where M = 2ml.
If l2 < < r2 then 
MAGNETIC POTENTIAL DUE TO A MAGNETIC POLE AT A POINT IN THE BROAD SIDE-ON POSITION.
Net potential at P = 0. The potential at any point lying on the magnetic equator of a magnet is zero in CGS and MKS system.
MAGNETIC POTENTIAL AT A POINT LYING ON A LINE PASSING THROUGH THE CENTRE AND MAKING ANGLE Θ WITH THE AXIS
EARTH’S MAGNETISM
Magnetic field of earth extends nearly upto five times the radius of earth i.e., 3.2 × 104 km.
The magnetic field of earth is fairly uniform and can be represented by equidistant parallel lines.
Geographic meridian : The geographic meridian at a place is the vertical plane passing through the geographic north & south at that place.
Magnetic meridian : The magnetic meridian at a place is the vertical plane passing through the magnetic axis of a freely suspended small magnet. The earth’s magnetic field acts in the plane of magnetic meridian.
ELEMENTS OF EARTH’S MAGNETIC FIELD
The earth’s magnetic field at a place can be completely described by three independent parameters called elements of earth’s magnetic field. These are :
- MAGNETIC DECLINATION (θ) : The angle between the geographic meridian and the magnetic meridian at a place is called the magnetic declination at that place.
- ANGLE OF DIP (δ) : The angle made by the earth’s total magnetic field with the horizontal is called angle of dip at any place.
True and apparent dip
When the plane of the dip circle is in the magnetic meridian, then the needle stops in the actual direction of the Earth's magnetic field. The angle made by the needle with the horizontal in this condition is called true dip.
In case, the plane of the dip circle is not in the magnetic meridian then the angle made by the needle with the horizontal is called apparent dip. In this case the vertical component of earth's magnetic field remains the same but the effective horizontal component B'H = BHcos θ
where δ′ = apparent dip
δ = true dip
If the dip circle is rotated by 90°, the new apparent dip δ′′, δ′ and δ are related as 
- HORIZONTAL COMPONENT OF EARTH’S MAGNETIC FIELD (BH) : It is the component of the earth’s total magnetic field in the horizontal direction and is given by, BH = B cos δ
KEEP IN MEMORY
- Dip circle is an instrument used to measure angle of dip at a place.
- At poles total magnetic intensity is 0.66 oersted and at equator it is 0.33 oersted
The total magnetic intensity at a particular latitude is 
where λ is the angle of latitude
The angle of dip δ = 0° at magnetic equator
and δ = 90° at magnetic poles.
- A spectacular effect due to earth's magnetism is observed near the magnetic poles of earth. This effect is called aurora-borealis in the north and aurora-australis in south. It is shown by patterns of coloured lights.
MAGNETIC MAPS
Magnetic surveys all over the earth have been carried out and magnetic maps have been prepared which show the values of magnetic element throughout the world. Lines can be drawn to join places having the same value of a particular magnetic element.
- Isogonic lines : These join places of equal declination. A line joining places of zero declination is called agonic line.
- Isoclinic lines : These join places of equal dip. A line joining the places of zero-dip is called aclinic line.
- Isodynamic lines : These join places of equal horizontal component.
Shielding from magnetic fields : For shielding a certain region of space from magnetic field, we surround the region by soft iron rings. Magnetic field lines will be drawn into the rings and the space enclosed will be free of magnetic field.
NEUTRAL POINTS
Neutral points are the points where the net field intensity due to the field of the bar magnet and field of earth is zero. When magnet is placed with its north pole towards geographic north, neutral points lie on equatorial line of the magnet. At each neutral point,
where H = horizontal component of earth’s magnetic field.
When the bar magnet is placed with its north pole towards geographic south, the neutral points lie on the axial line of the magnet. At each neutral point,
RELATION BETWEEN THE UNITS OF QUANTITIES ASSOCIATED WITH MAGNETIC FIELD
1A = 1JT–1 m–2 = 1J Wb–1
1T = 1JA–1 m–2 = 1Wb m–2
1Wb = 1JA–1 = 1 Tm2
[B] = NA–1 m–1 = T = Wb m–2
[M] = A m2 = JT–1 = J m2 Wb–1
[μ0] = NA–2 = T2 m2 N–1 = Wb2 J–1 m–1
1Wb = 1JA–1 = 1 Tm2
[B] = NA–1 m–1 = T = Wb m–2
[M] = A m2 = JT–1 = J m2 Wb–1
[μ0] = NA–2 = T2 m2 N–1 = Wb2 J–1 m–1
TERMS RELATED TO MAGNETISM
MAGNETIC INTENSITY (H)
When a magnetic material is placed in a magnetic field, it becomes magnetised. The capability of the magnetic field to magnetise a material is expressed by means of a magnetic vector  , called the `magnetic intensity’ of the field. The relation between magnetic induction B and magnetising field
, called the `magnetic intensity’ of the field. The relation between magnetic induction B and magnetising field 
 , μ being permeability of medium.
, μ being permeability of medium.
INTENSITY OF MAGNETISATION (I)
When a material is placed in a magnetising field, it acquires magnetic moment M. The intensity of magnetisation is defined as the magnetic moment per unit volume i.e.,

V being volume of material. If the material is in the form of a bar of cross-sectional area A, length 2l and pole strength m, then
M = m × 2l; V = A × 2l

MAGNETIC SUSCEPTIBILITY (χ) : The magnetic susceptibility is defined as the intensity of magnetisation per unit magnetising field. i.e.,

MAGNETIC PERMEABILITY (μ) : The magnetic permeability of a material is the measure of degree to which the material can be permitted by a magnetic field and is defined as the ratio of magnetic induction (B) in the material to the magnetising field i.e.

RELATION BETWEEN MAGNETIC SUSCEPTIBILITY AND PERMEABILITY
We have magnetic induction in material, B = μH
Also B = B0 + Bm
where B0 = magnetic induction in vacuum produced by magnetising field
Bm = magnetic induction due to magnetisation of material.
But B0 = μ0H and Bm = μ0m Im ⇒ 
This is required relation.
CLASSIFICATION OF MATERIALS
According to the behaviour of substances in magnetic field, they are classified into three categories:
DIAMAGNETIC SUBSTANCES
These are the substances which when placed in a strong magnetic field acquire a feeble magnetism opposite to the direction of magnetising field.
Examples are copper, gold, antimony, bismuth, alcohol, water, quartz, hydrogen, etc.
Behaviour of diamagnetic substance in an external magnetic field 
CHARACTERISTICS OF DIAMAGNETIC SUBSTANCES
- They are feebly repelled by a strong magnet
- Their susceptibility is negative (i.e. χ < 0)
- Their relative permeability is less than 1. (i.e. μr < 1)
- Their susceptibility is independent of magnetising field and temperature (except for Bismuth at low temperature)
PARAMAGNETIC SUBSTANCES
These are the materials which when placed in a strong magnetic field acquire a feeble magnetism in the same sense as the applied magnetic field.
Examples are platinum, aluminium, chromium, manganese, CuSO4, O2, air, etc.
Behaviour of paramagnetic substance in an external field 
CHARACTERISTICS OF PARAMAGNETIC SUBSTANCES
- They are attracted by a strong magnet
- Their susceptibility is positive but very small (χ > 0)
- Their relative permeability is slightly greater than unity. (μ > 1)
- Their susceptibility and permeability do not change with the variation of magnetising field.
- Their susceptibility is inversely proportional to temperature,
- They are found in those material which have atoms containing odd number of electrons.
FERROMAGNETIC SUBSTANCES
These are the substances which are strongly magnetised by relatively weak magnetising field in the same sense as the magnetising field.
Examples are Ni, Co, iron and their alloys.
CHARACTERISTICS OF FERROMAGNETIC SUBSTANCES
- They are attracted even by a weak magnet.
- The susceptibility is very large and positive. (χ >> 0)
- The relative permeability is very high (of the order of hundreds and thousands). (μ >> 1)
- The intensity of magnetisation is proportional to the magnetising field H for smaller values, varies rapidly for moderate values and attains a constant value for larger values of H.
- The susceptibility of a ferromagnetic substance is inversely proportional to temperature i.e.,
This is called Curie law. At a temperature called curie temperature, ferromagnetic substance becomes paramagnetic. The curie temperatures for Ni, Fe and Co are 360ºC, 740ºC and 1100ºC respectively.
- They are found in those material which have domains and can be converted into strong magnets
KEEP IN MEMORY
- Diamagnetism is universal. It is present in all materials. But it is weak and hard to detect if substance is para or ferromagnetic.
- I – H curve for different materials
- Curve for magnetic susceptibility and temperature for a paramagnetic and ferromagnetic material.
HYSTERESIS
When a bar of ferromagnetic material is magnetised by a varying magnetic field and the intensity of magnetisation Im induced is measured for different values of magnetising field H, the graph of I versus H is as shown in fig
The graph shows :
- When magnetising field is increased from O the intensity of magnetisation m increases and becomes maximum (i.e. point A). This maximum value is called the saturation value.
- When H is reduced, m reduces but is not zero when H = 0. The remainder value OB of magnetisation when H = 0 is called the residual magnetism or retentivity. OB is retentivity.
- When magnetic field H is reversed, the magnetisation decreases and for a particular value of H, it becomes zero i.e., for H = OC, I = 0. This value of H is called the coercivity.
- When field H is further increased in reverse direction, the intensity of magnetisation attains saturation value in reverse direction (i.e., point D).
- When H is decreased to zero and changed direction in steps, we get the part DFGA.
PROPERTIES OF SOFT IRON AND STEEL
For soft iron, the susceptibility, permeability and retentivity are greater while coercivity and hysteresis loss per cycle are smaller than those of steel.
PERMANENT MAGNETS AND ELECTROMAGNETS
Permanent magnets are made of steel and cobalt while electromagnets are made of soft iron.
An electromagnet is made by inserting a soft iron core into the interior of a solenoid. Soft iron does not retain a significant permanent magnetization when the solenoid’s field is turned off–soft iron does not make a good permanent magnet. When current flows in the solenoid, magnetic dipoles in the iron tend to line up with the field due to the solenoid. The net effect is that the field inside the iron is intensified by a factor known as the relative permeability. The relative permeability is analogous in magnetism to the dielectric constant in electricity. However, the dielectric constant is the factor by which the electric field is weakened, while the relative permeability is the factor by which the magnetic field is strengthened. The reactive permeability of a ferromagnet can be in the hundreds or even thousands–the intensification of the magnetic field is significant. Not only that, but in an electromagnet the strength and even direction of the magnetic field can be changed by changing the current in the solenoid.
KEEP IN MEMORY
- By alloying soft-iron with 4% silicon ‘transformer steel’ is produced. It has a higher relative permeability and is an ideal material for cores of transformers. Alloys of iron and nickel called ‘permalloys’, also have very large permeabilities.
- Energy spent per unit volume of specimen is complete cycle of magnetisation is numerically equal to area of I – H loop
Steel is most suitable for making permanent magnet
Soft iron is most suitable for making core of an electromagnet.
TANGENT GALVANOMETER
It is an instrument used for measuring small current. It is based on tangent law. It is a moving magnet and fixed coil type galvanometer.
TANGENT LAW
If a small magnetic needle is under the influence of two crossed magnetic fields (B) and (H) and suffers a deflection θ from field H, then by tangent law, B = H tan θ.
FORMULA FOR CURRENT
If a current passing through the coil of n turns and mean radius r of a tangent galvanometer placed in magnetic meridian causes a deflection θ in the magnetic needle kept at the centre of the coil, then
where  and is called the reduction factor.
and is called the reduction factor.
DEFLECTION MAGNETOMETER
It's working is based on the principle of tangent law.
Tan A Position
In this position the magnetometer is set perpendicular to magnetic meridian so that, magnetic field due to magnet is in axial position and perpendicular to earth’s field and hence
where d = distance of needle from centre of magnet and 2l = length of magnet.
TanB position
The arms of magnetometer are set in magnetic meridian, so that the field is at equatorial position and hence,

Magnetic field of earth extends nearly upto five times the radius of earth i.e., 3.2 × 104 km.
The magnetic field of earth is fairly uniform and can be represented by equidistant parallel lines.
VIBRATION MAGNETOMETER
It is an instrument for comparing the magnetic moments of two magnets and for comparing their magnetic fields.
The time period of a bar magnet vibrating in the vibration magnetometer kept in magnetic meridian is given by
where  is the moment of inertia of the vibrating magnet,
is the moment of inertia of the vibrating magnet,
m = mass of magnet,
l = length of magnet,
b = breadth of magnet.
m = mass of magnet,
l = length of magnet,
b = breadth of magnet.










