Complex Number and Quadratic Equations Quiz-24
Assertion - Reasoning Type
Dear Readers,
Complex numbers and quadratic equations is a segment of maths that deals
with crucial theorems and concepts along with various formulae. It
comprises of linear and quadratic equations along with roots related to
the complex number's set (known as complex roots)..
Q1.
Statement 1: The greatest integral value of λ for which (2λ-1)
x2-4x+(2λ-1)=0 has real roots, is 2.
Statement 2: For real roots of ax2+bx+c=0,D≥0.
Statement 2: For real roots of ax2+bx+c=0,D≥0.
Solution
Q2. Statement 1: If roots of the equation x2-bx+c=0 are
two consecutive integers, then b2-4c=1
Statement 2: If a,b,c are odd integer, then the roots of the equation 4 abc x2+(b2-4ac)x-b=0 are real and distinct
Statement 2: If a,b,c are odd integer, then the roots of the equation 4 abc x2+(b2-4ac)x-b=0 are real and distinct
Solution
Q3. Let ax2+bx+c=0,a≠0 (a,b,c∈R) has no real roots and
a+b+2c=2
Statement 1: ax2+bx+c>0,∀ x∈R
Statement 2: a+b is positive
Statement 1: ax2+bx+c>0,∀ x∈R
Statement 2: a+b is positive
Solution
Q4. Statement 1: If cos(1-i)=a+ib,where a,b∈R and i=√(-1),then a=1/2 (e+1/e) cos1,b=1/2 (e-1/e)sin1
Statement 2: eiθ=cosθ+isinθ
Solution
Q5. Let fourth roots of unity z1,z2,z3
and z4 respectively
Statement 1: z12+z22+z32+z42=0
Statement 2: z1+z2+z3+z4=0
Statement 1: z12+z22+z32+z42=0
Statement 2: z1+z2+z3+z4=0
Solution
Q6. Statement 1: The equation (x-p)(x-r)+λ(x-q)(x-s)=0, where
p<q<r<s, has non-real roots
Statement 2: The equation px2+qx+r=0 (p,q,r∈R) has non-real roots if q2-4pr<0
Statement 2: The equation px2+qx+r=0 (p,q,r∈R) has non-real roots if q2-4pr<0
Solution
Q7. Statement 1: If both roots of the equation
2x2-x+a=0(a∈R) lies in (1, 2), then-1<a≤1/8.
Statement 2: If F(x)=2x2-x+a, then D≥0,f(1)>0,f(2)>0 yield-1<a≤1/8.
Statement 2: If F(x)=2x2-x+a, then D≥0,f(1)>0,f(2)>0 yield-1<a≤1/8.
Solution
Q8. Statement 1: The number of values of a for which
(a2-3a+2)
x2+(a2-5a+6)x+a2-4=0 is an
identity in x is 2
Statement 2: If a=b=c=0, then equation ax2+bx+c=0 is an identity in x
Statement 2: If a=b=c=0, then equation ax2+bx+c=0 is an identity in x
Solution
Q9.
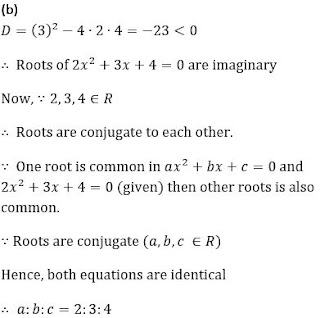
Statement 1: If equations ax2+bx+c=0,(a,b,c∈R) and
2x2+3x+4=0 have a common root, then a∶b∶c=2∶3∶4.
Statement 2: Roots of 2x2+3x+4=0 are imaginary.
Statement 2: Roots of 2x2+3x+4=0 are imaginary.
Solution
Q10. Statement 1: If z1 and z2 are two complex
numbers such that |z1 |=|z2
|+|z1-z2 |, then Im (z1/z2
)=0
Statement 2: arg(z)=0⇒z is purely real
Statement 2: arg(z)=0⇒z is purely real
Solution
