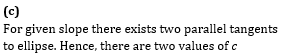Conic Section Quiz-31
Dear Readers,
In JEE exams, Conic section is one of the most important topic of Mathematics that comes under Coordinate Geometry, it has total of 15 percent weightage out of which 6% questions are asked in JEE mains and 9% in Advance.
Q1.
The number of values of c such that the straight line y=4x+c touches the curve x^2/4+y^2/1=1 is
Solution
Q2.
Let S be the focus of y^2=4x and a point P is moving on the curve such that its abscissa is increasing at the rate of 4 units/sec, then the rate of increase of projection of SP on x+y=1 when P is at (4,4) is
Q3.
The locus of the centre of a circle which cuts orthogonally the parabola y^2=4x at (1, 2) will pass though points
Solution
Q4. The area of the triangle formed by the tangent and the normal to the parabola y^2=4ax, both drawn at the same end of the latus rectum, and the axis of the parabola is
Solution
Q5.
A square is inscribed in the circle x^2+y^2-2x+4y-93=0 with its sides parallel to the coordinate axis. The co-ordinates of its vertices are
Solution
Q6.
A normal to the hyperbola x^2/4-y^2/1=1 has equal intercepts on positive x- and y-axes. If this normal touches the ellipse x^2/a^2 +y^2/b^2 =1, then a^2+b^2 is equal to
- 5
- 25
- 16
- None of these
Solution
Q7.
If the tangents are drawn from any point on the line x+y=3 to the circle x^2+y^2=9, then the chord of contact passes through the point
Q8.
A straight line has its extremities on two fixed straight lines and cuts off from them a triangle of constant area c^2. Then the locus of the middle point of the line is
Solution
Q9.
If the normals to the parabola y^2=4ax at three points P,Q and R meet at A and S be the focus, then SP.SQ.SR is equal to
Solution
Q10.
If the line x cos〖θ+y sin〖θ=2〗 〗 is the equation of a transverse common tangent to the circles x^2+y^2=4 and x^2+y^2-6√3 x-6y+20=0, then the value of θ is
Solution
