Conic Section Quiz-33

Conic Section Quiz-33

Dear Readers,

In JEE exams, Conic section is one of the most important topic of Mathematics that comes under Coordinate Geometry, it has total of 15 percent weightage out of which 6% questions are asked in JEE mains and 9% in Advance.

Q1.  Two circles x^2+y^2=6 and x^2+y^2-6x+8=0 are given. Then, the equation of the circle through their points of intersection and the point (1, 1) is

•   x^2+y^2-6x+4=0
•   x^2+y^2-3x+1=0
•   x^2+y^2-4y+2=0
•   None of these

Solution

Q2.  The number of rational point(s) (a point (a,b) is called rational, if a and b both are rational numbers) on the circumference of a circle having centre (π,e) is

•  At most one
•  At least two
•  Exactly two
•  Infinite

Q3.  A tangent drawn to hyperbola x^2/(a^2 )-y^2/(b^2 )=1 at P(π/6) forms a triangle of area 3a^2 square units, with coordinate axes, then the square of its eccentricity is

•   15
•   24
•   17
•   14

Solution

Q4.    With a given point and line as focus and directrix, a series of ellipses are described, the locus of the extremities of their minor axis is

•   Ellipse
•   Parabola
•   Hyperbola
•   None of these

Solution

Q5.  The combined equation of the asymptotes of the hyperbola 2x^2+5xy+2y^2+4x+5y=0 is

•  2x^2+5xy+2y^2+4x+5y+2=0
•  2x^2+5xy+2y^2+4x+5y-2=0
•  2x^2+5xy+2y^2=0
•  None of these

Solution

Q6.  A tangent and normal is drawn at the point P≡(16,16) of the parabola y^2=16x which cut axis of the parabola at the points A and B, respectively. If the centre of the circle through P,A and B is C, then the angle between PC and the axis of is

•   tan^(-1)⁡〖1/2〗
•   tan^(-1)⁡2
•   tan^(-1)⁡〖3/4〗
•   tan^(-1)⁡〖4/3〗
Solution

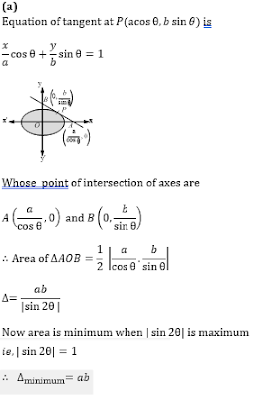
Q7.  Tangent at a point of the ellipse x^2/a^2 +y^2/b^2 =1 is drawn which cuts the coordinate axes at A and B. The minimum area of the ∆OAB is (O being the origin)

•   ab
•   (a^3+ab+b^3)/3
•  a^2+b^2
•   ((a^2+b^2))/4

Q8.  Two circles of radii 4 cm and 1 cm touch each other externally and θ is the angle contained by their direct common tangents. Then sin⁡θ is equal to

•  24/25
•  12/25
•  3/4
•  None of these

Solution

Q9. Four points are such that the line joining any two points is perpendicular to the line joining other two points. If three points out of these lie on a rectangular hyperbola then the fourth point will lie on

•   The same hyperbola
•   Conjugate hyperbola
•   One of the directrix
•   One of the asymptotes

Solution

Q10. A point P(x,y) moves in xy plane such that x=a cos^2⁡θ and y=2a sin⁡θ, where θ is a parameter. The locus of the point P is

•  Circle
•  Parabola
•  Unbounded parabola
•  Part of the parabola

Solution