Conic Section Quiz-35

Conic Section Quiz-35

Dear Readers,

In JEE exams, Conic section is one of the most important topic of Mathematics that comes under Coordinate Geometry, it has total of 15 percent weightage out of which 6% questions are asked in JEE mains and 9% in Advance.

Q1.  If point A and B are (1, 0) and B(0,1). If point C is on the circle x^2+y^2=1, then locus of the orthocenter of the triangle ABC is

•   x^2+y^2=4
•   x^2+y^2-x-y=0
•   x^2+y^2-2x-2y+1=0
•   x^2+y^2+2x-2y+1=0

Solution

Q2.  A man running round a race course notes that the sum of the distances of two flagposts from him is always 10 m and the distance between the flag posts is 8 m. Then the area of the path he encloses in square meters is

•  15π
•  20π
•  27π
•  30π

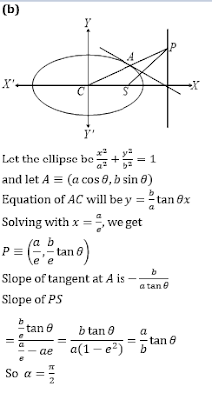
Q3.  Let P be any point on a dirextrix of an ellipse of eccentricity e. S be the corresponding focus and C the centre of the ellipse. The line PC meets the ellipse at A. The angel between PS and tangent at A is α, the α is equal to

•   tan^(-1)⁡e
•   π/2
•   tan^(-1) (1-e^2 )
•   None of these

Solution

Q4.    Equation of incircle of equilateral triangle ABC where B≡(2,0)C≡(4,0) and A lies in fourth quadrant is

•   x^2+y^2-6x+2y/√3+9=0
•   x^2+y^2-6x-2y/√3+9=0
•   x^2+y^2+6x+2y/√3+9=0
•   None of these

Solution

Q5.  Axis of a parabola is y=x and vertex and focus are at a distance √2 and 2√2 Respectively from the origin. Then, equation of the parabola is

•  (x-y)^2=8(x+y-2)
•  (x+y)^2=2(x+y-2)
•  (x-y)^2=4(x+y-2)
•  (x+y)^2=2(x-y+2)

Solution

Q6.  The equation of the parabola whose focus is the point (0,0) and the tangent at the vertex is x-y+1=0 is

•   x^2+y^2-2xy-4x-4y-4=0
•   x^2+y^2-2xy+4x-4y-4=0
•   x^2+y^2+2xy-4x+4y-4=0
•   x^2+y^2+ 2xy-4x-4y+4=0
Solution

Q7.  If tangents PQ and PR are drawn from variable point P to the hyperbola x^2/a^2 -y^2/b^2 =1(a>b) so that the fourth vertex S of parallelogram PQSR lies on circumcircle of triangle PQR, then locus of P is

•   x^2+y^2=b^2
•   x^2+y^2=a^2
•  x^2+y^2=(a^2-b^2)
•   None of these

Q8.  Locus of the point √(3h,) √(3k+2 ) if it lies on the line x-y-1=0 is a

•  Straight line
•  Circle
•  Parabola
•  None of these

Solution

Q9. Asymptotes of the hyperbola x^2/(a_1^2 )-y^2/(b_1^2 )=1 and x^2/(a_2^2 )-y^2/(b_2^2 )=1 are perpendicular to each other, then

•   a_1/a_2 =b_1/b_2
•   a_1 a_2=b_1 b_2
•   a_1 a_2+b_1 b_2=0
•   a_1-a_2=b_1-b_2

Solution

Q10. The set of values of m for which it is possible to draw the chord y=√m x+1 to the curve x^2+2xy+(2+sin^2⁡α ) y^2=1, which subtends a right angle at the origin for some value of α is

•  [2,3]
•  [0,1]
•  [1,3]
•  None of these

Solution