Permutations and Combinations is one of the most important chapters of Algebra in the JEE syllabus and other engineering exams. For JEE Mains, it has 4% weightage and for JEE Advanced, it has 5% weightage..
This section contain(s) 10 questions numbered 1 to 10. Each question contains statement 1(Assertion) and statement 2(Reason). Each question has the 4 choices (a), (b), (c) and (d) out of which only one is correct.
a)Statement 1 is True, Statement 2 is True; Statement 2 is correct explanation for Statement 1
b)Statement 1 is True, Statement 2 is True; Statement 2 is not correct explanation for Statement 1
c)Statement 1 is True, Statement 2 is False
d)Statement 1 is False, Statement 2 is True
.
a)Statement 1 is True, Statement 2 is True; Statement 2 is correct explanation for Statement 1
b)Statement 1 is True, Statement 2 is True; Statement 2 is not correct explanation for Statement 1
c)Statement 1 is True, Statement 2 is False
d)Statement 1 is False, Statement 2 is True
.
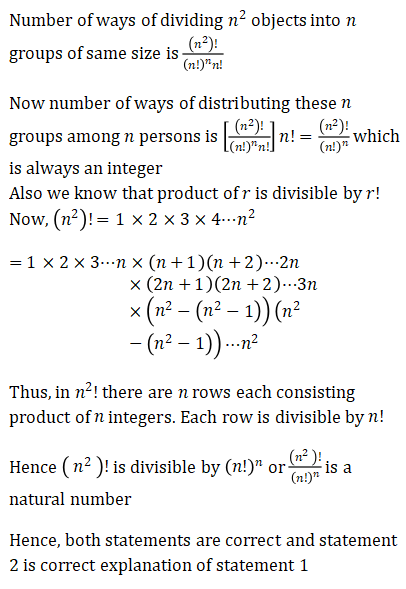
Q1. Statement 1: (𝑛2)!/(𝑛!)𝑛 is a natural number for all 𝑛 ∈ 𝑁
Statement 2: Number of ways in which 𝑛2objects can be distributed among 𝑛 persons equally is (𝑛2)!/(𝑛!)𝑛
Q2.In a shop there are five types of ice-creams available. A child buys six ice-creams
Statement 1: The number of different ways the child can buy the six ice-creams is 10𝐶5
Statement 2: The number of different ways the child can buy the six ice-creams is equals to the number of different ways of arranging 6 A’s and B’s in a row.
Q3. Statement 1: Number of ways in which 30 can be partitioned into three unequal parts, each part being a natural number is 61
Statement 2: Number of ways of distributing 30 identical objects in three different boxes is 30𝐶2
Q4. Statement 1: Number of ways in which Indian team (11 players) can bat, if Yuvraj wants to bat before Dhoni and Pathan wants to bat after Dhoni is 11!/3! Statement 2: Yuvraj, Dhoni and Pathan can be arranged in batting order in 3! Ways
Q5. Statement 1: Number of zeros at the end of 50! Is equal to 12
Statement 2: Exponent of 2 in 50! is 47
Q6. Statement 1: The number of non-negative integral solutions of 𝑥1+𝑥2+𝑥3+...+𝑥𝑛 = 𝑟 is 𝑟+𝑛−1𝐶𝑟
Statement 2: The number of ways in which 𝑛 identical things can be distributed into 𝑟 different groups is 𝑛+𝑟−1𝐶𝑛
Q7.Statement 1: The number of ways in which 𝑛 persons can be seated at a round table, so that all shall not have the same neighbours in any two arrangements is (𝑛−1)!/2
Statement 2: Number of ways of arranging 𝑛 different beads in circles in which is (𝑛−1)!/2
Q8.Statement 1: Number of ways of selecting 10 objects from 42 objects of which, 21 objects are identical and remaining objects are distinct is 220
Statement 2: 42𝐶0+ 42𝐶1+ 42𝐶2+⋯+ 42𝐶21 = 241
Q9.Statement 1: Number of ways in which 10 identical toys can be distributed among 3 students, if each receives atleast one toys is 9𝐶2
Statement 2: Number of positive integral solutions of 𝑥 + 𝑦 + 𝓏 + 𝑤 = 7 is 6𝐶2
Q10.Statement 1: When number of ways of arranging 21 objects of which 𝑟 objects are identical of one type and remaining are identical of second type is maximum, then maximum value of 13𝐶𝑟 is 78
Statement 2: 2𝑛+1𝐶𝑟 is maximum when 𝑟 = 𝑛