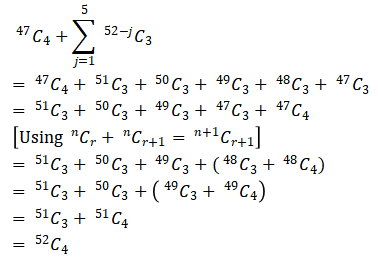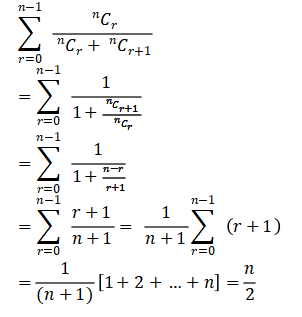Permutations and Combinations-Quiz-4

Permutations and Combinations Quiz-4

Dear Readers,

Permutations and Combinations is one of the most important chapters of Algebra in the JEE syllabus and other engineering exams. For JEE Mains, it has 4% weightage and for JEE Advanced, it has 5% weightage..

Q1. In how many ways can 17 persons depart from railway station in 2 cars and 3 autos, given that 2 particular persons depart by same car (4 persons can sit in a car and 3 persons can sit in an auto)?

•  15!/(2!4!(3!)³)
•  16!/((2!)²4!(3!)³)
•  17!/(2!4!(3!)³)
•  15!/(4!(3!)³)

Solution

Q2.The number of ways in which 12 books can be put in the three shelves with four on each shelf is

•  12!/(4!)³
•  12!/(3!(4!)³)
•  12!/((3!)³4!)
•  None of these

Solution
Since the shelves which are to receive the books are different, therefore the required number of ways is 12!/(4!)³

Q3.  Let A be a set of n (>e;3) distinct elements. The number of triplets (x,y,z) of the A elements in which at least two coordinates is equal to

•  ⁿP₃
•  n³ - ⁿP₃
•  3n² - 2n
•  3n²(n - 1)

Solution
Total number of triplets without restriction is n x n x n. The number of triplets with all different coordinates is ⁿP₃

Q4. A bag contains four one-rupee coins, two twenty-five paisa coins and five ten-paisa coins. In how many ways can an amount, not less then Re 1 be taken out from the bag? (consider coins of the same denominations to be identical)

•  71
•  72
•  73
•  80

Solution
When at least one one-rupee coin is selected we can select any number of twenty five coins and ten paisa coins. Then number of ways of such selection is 4(2 + 1)(5 + 1) = 72 as we can select zero or more twenty five paisa and ten paisa coins to ensure that amount selected is Re. 1 or more.
But when none of one-rupee coins is selected we have to select all twenty five paisa coins and ten paisa coins to ensure sum of Re. 1, which can be done only in one way. Then the total number of ways is 73

Q5.The number of ways to give 16 different things to three persons A,B,C so that B gets one more than A and C gets two more than B, is

•  16!/(4!5!7!)
•  4!5!7!
•  16!/(3!5!8!)
•  None of these

Solution
Obviously, A, B and C get 4, 5 and 7 objects, respectively. Then, number of distribution ways is equal to number of division of ways, which is given by 16!(4!5!7!)

Q6. A man has three friends. The number of ways he can invite one friend everyday for dinner on six successive nights so that no friend is invited more than three times is

•  640
•  320
•  420
•  510

Solution

Q7.There are 10 points in a plane of which no three points are collinear and four points are concyclic. The number of different circles that can be drawn through at least three points of these points is

•  116
•  120
•  117
•  None of these

Solution
Number of points required for the fixed circle is 3. So, first select any three points from the 10 points in ¹⁰C₃ ways. In these ways, circle with four concyclic points is selected in ⁴C₃ ways. But is should be taken once then total number of circles is (¹⁰C₃ - ⁴C₃) + 1

Q8.How many numbers can be made with the digits 3, 4, 5, 6, 7, 8 lying between 3000 and 4000, which are divisible by 5 while repetition of any digit is not allowed in any number?

•  60
•  12
•  120
•  24

Solution
3 must be at thousand’s place and since the number should be divisible by 5, or 5 must be at unit’s place. Now, we have to fill two places (tens and hundreds), i.e., ⁴P₂ = 12

Q9. 

•  ⁴⁷C₅
•  ⁵²C₅
•  ⁵²C₄
•  None of these

Solution

Q10. 

•  n + 1
•  n/2
•  n + 2
• None of these

Solution