JEE Advanced Physics Syllabus can be referred by the IIT aspirants to get a detailed list of all topics that are important in cracking the entrance examination. JEE Advanced syllabus for Physics has been designed in such a way that it offers very practical and application-based learning to further make it easier for students to understand every concept or topic by correlating it with day-to-day experiences. In comparison to the other two subjects, the syllabus of JEE Advanced for physics is developed in such a way so as to test the deep understanding and application of concepts.</.
Q1. Image of an object approaching a convex mirror of radius of curvature 20 m along its optical axis is observed to move from 25/3 m to 50/7 m in 30 s. What is the speed of the object in kmh⁻¹?
Solution
(a) Using mirror formula, 1/(+25/3)+ 1/(-u₁) = 1/(+10)
Or 1/u_1 = 3/25 - 1/10
Or u_1 = 50 m And 1/((+50/7) )+ 1/(-u_1 ) = 1/(+10)
∴1/u_2 = 7/50 - 1/10 Or u_2 = 25 m
Speed of object =(u_1- u_2)/time =25/30 ms^(-1) = 3 kmh^(-1)
(a) Using mirror formula, 1/(+25/3)+ 1/(-u₁) = 1/(+10)
Or 1/u_1 = 3/25 - 1/10
Or u_1 = 50 m And 1/((+50/7) )+ 1/(-u_1 ) = 1/(+10)
∴1/u_2 = 7/50 - 1/10 Or u_2 = 25 m
Speed of object =(u_1- u_2)/time =25/30 ms^(-1) = 3 kmh^(-1)
Q2.A convex spherical refracting surface with radius R separates a medium having refractive index 5/2 from air. As an object is moved towards the surface from far away from the surface along the principle axis, its image
Q3. A convex lens of focal length 1.0 m and a concave lens of focal length 0.25 m are 0.75 m apart. A parallel beam of light is incident on the convex lens. The beam emerging after refraction from both lenses is
Solution
Power of system
1/f_1 +1/f_2 -d/(f_1 f_2 )=1/1+1/(-0.25)-0.75/(1)(-0.25)
Since power of the system is zero, therefore the incident parallel beam of light will remain parallel after emerging from the system
Power of system
1/f_1 +1/f_2 -d/(f_1 f_2 )=1/1+1/(-0.25)-0.75/(1)(-0.25)
Since power of the system is zero, therefore the incident parallel beam of light will remain parallel after emerging from the system
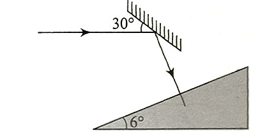
Q4. Find the net deviation produced in the incident ray for the optical instrument shown in figure below. (Take refractive index of the prism material as 2.)
Q5. Two point sources S_1 and S_2 are 24 cm apart. Where should a convex lens of focal length 9 cm be placed in between them so that the image of both sources are formed at the same place?
Q6. A hallow double concave lens is made of very thin transparent material. It can be filled with air or either of two liquids L_1 or L_2 having refractive indices n_1 and n_2, respectively (n_2>n_1>1). The lens will diverge parallel beam of light if it is filled with
Solution
If the refractive index of the material of the lens is greater than the refractive index of the surrounding medium, then a concave lens would behave as a concave lens
If the refractive index of the material of the lens is greater than the refractive index of the surrounding medium, then a concave lens would behave as a concave lens
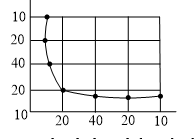
Q7. A lens forms a real image of an object. The distance from the object to the lens is x cm and that from the lens to the image is y cm. the graph (see figure) shows the variation of y with x It can be deduced that the lens is
Solution
A diverging lens is ruled out because both x and y are positive values. Both x and y equal 20 cm at their smallest sum, which occurs when
x+y=40 cm=4f
∴f=10 cm
This indicates a converging lens of focal length =10 cm
A diverging lens is ruled out because both x and y are positive values. Both x and y equal 20 cm at their smallest sum, which occurs when
x+y=40 cm=4f
∴f=10 cm
This indicates a converging lens of focal length =10 cm
Q8.Figure shows the graph of angle of deviation δ versus angle of incidence i for a light ray striking a prism. The prism angle is
Solution
δ=e+i-A
30°=15°+60°-A
⇒A=45°
δ=e+i-A
30°=15°+60°-A
⇒A=45°
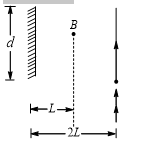
Q9. A point source of light B is placed at a distance L in front of the center of a mirror of width d hung vertically on a wall. A man walks in front of the mirror along a line parallel to the mirror at a distance 2L from it as shown in figure. the greatest distance over which he can see the image of the light source in the mirror is
Q10. If ε_0 and μ_0 are respectively, the electric permittivity and the magnetic permeability of free space, ε and μ the corresponding quantities in a medium, the refractive index of the medium is
Solution
μ=c/v=(1/√(μ_o ε_o ))/(1/√με)=√(με/(μ_o ε_o ))
μ=c/v=(1/√(μ_o ε_o ))/(1/√με)=√(με/(μ_o ε_o ))