Work Energy and Power-Quiz-10

WORK ENERGY AND POWER Quiz-10

Dear Readers,

Work power energy is the most important chapter when it comes to mechanics, for JEE (Advanced). The chapter is quite tricky and takes a lot of time and devotion on your part to understand and master. But this chapter is a complete gold medal for almost all the questions of the mechanics can be solved by the work power energy approach if you master this topic..

Q1.

•  Block C will move for a very small duration
•  Block A will move for a very small duration
•  Block B will acquire maximum speed when C is at the lowest point on B and moving towards left
•  Block B will acquire maximum speed when C is at the topmost point of B

Solution

When block C is released from rest, it sides down on A, pushing it against the wall (because of a component of normal force between A and C), as on left of A a wall is there, it does not move. The kinetic energy of block C incrases and it becomes mgh when it is just going to pass on B When block C comes in contact with B, sue to horizontal component of normal force between C and B,B starts moving towards right and kinetic energy of C gets converted into kinetic energy of B and potential energy of C, so block C is not able to reach the topmost point of B. Then block C starts sliding down B, but velocity of B will still be increasing and becomes maximum when C reaches its bottom-most point and is moving left

Q2.

•  1/2 kx^2
•  1/2 (kx)(s+x)
•  1/2 kxs
•  1/2 kx(s+x+s)

Solution

Total work done is equal to the energy stored in the spring

Q3.  

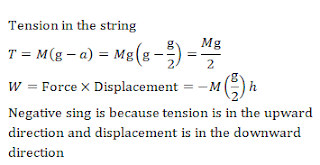
A mass M is lowered with the help of a string by a distance h at a constant acceleration g/2. The work done by the string will be

•  Mgh/2
•  -Mgh/2
•  3Mgh/2
•  -3Mgh/2

Solution

Q4. 

•  R[2+1/(2√2)]
•  [1+1/(2√2)]R
•  3R
•  None of these

Solution

Q5.

•  mg/(4k)
•  4mg/k
•  mg/(2k)
•  None

Solution
 

Q6. 

A weightlifter lifts a weight off the ground and holds it up, then

•  Work is done in lifting as well as holding the weight
•   No work is done in both lifting and holding the weight
• Work is done in lifting the weight but no work is required to be done in holding it up
•  No work is done in lifting the weight but work is required to be done in holding it up

Solution

When a weightlifter lifts a weight by height h (say), then work done by the lifting force F W1=RFs cos⁡ 0°=+Fh But work done in holding it up is zero because the displacement is zero

Q7.

Water is drawn from a well in a 5 kg drum of capacity 55 L by two ropes connected to the top of the drum. The linear mass density of each rope is 0.5 kgm^(-1). The work done in lifting water to the ground from the surfaces of water in the well 20 m below is [g=10 ms^(-2)]

•  1.4×10^4 J
•  1.5×10^4 J
•  9.8×10^6 J
•  18 J

Solution

Work done in lifting water and drum is 60×10×20 J=12000 J
Total mass of ropes =40×0.5 kg=20 kg
Work done in the case of ropes is 20×10×10=2000 J
Total work done=14000 J

Q8.

Which of the following statements is correct?

•  Kinetic energy of a system can be changed without changing its momentum
•  Kinetic energy of a system cannot be changed without changing its momentum
•  Momentum of a system cannot be changed without changing its kinetic energy
•  A system cannot have energy without having momentum

Solution

In the explosion of a bomb or inelastic collision between two bodies as force is internal, momentum is conserved while KE changes. Hence, the KE of a system can be changed without changing its momentum. Similarly, the reverse is also true, e.g., if a force acts perpendicular to motion, work done will be zero and so KE will remain constant. However, the force will change the direction of motion and so the momentum. Further, body may have energy (i.e., potential energy without having momentum)

Q9. 

•  Increase with θ
•  Decrease with θ
•  Remain constant
•  First increase with θ and then decrease

Solution

Q10. 

In a tug of war, both the teams A and B remain in equilibrium, then

•  Work done by team A is positive
•  Work done by team B is positive
•  Work done by both the teams is negative
• Work done by both the teams is zero

Solution

Because both the teams balance each other, displacement is zero. Therefore, work done is also zero